Resolve two forces at 120º to each other?
2 Answers
Resolving the non-horizontal force and adding the components to the horizontal force shows that the answer is 10 N.
(This is one way of solving.)
Explanation:
If we resolve the non-horizontal force into a horizontal and vertical component we can add those to the horizontal force by vector addition to solve the problem.
First resolve the non-horizontal force into horizontal and vertical components:
We can divide the total 120º angel neatly around a vertical line from P. To the right of the vertical is a 90º angle to the horizontal force, therefore from the vertical to the other force is an angle of 30º.

So the vertical component,
And the horizontal component,
Now add the horizontal component to the horizontal force:
The forces are in opposite directions so they subtract. Taking to the right as positive, the resultant of these two forces is
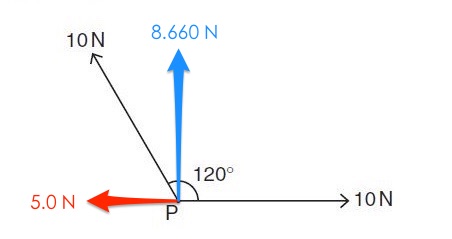
So the total horizontal force is 5.0 N to the right (shown in green in the next diagram).

The last step is to calculate the magnitude of the resultant of these two forces:
The total horizontal force and the vertical component form a right angled triangle, so we can use Pythagoras’ Theorem.
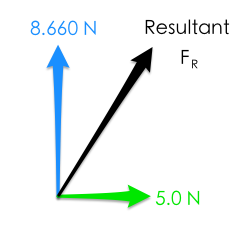
You can use the cosine rule to solve the problem if you rearrange the forces head-to-tail.
Explanation:
If you want a more elegant solution than the one I just provided, well here it is!
We can rearrange the problem so that the two forces are arranged head-to-tail and use the cosine rule to solve for the resultant force.
Rearrange the forces so they are head-to-tail:
The diagram shows the starting arrangement and the new arrangement with the forces head-to-tail. The new arrangement also shows the resultant force (that starts at P and ends where the second 10 N force ends). The 120º angle is shown for reference. We also know that the angle between the 10 N forces is 60º since 180º – 120º = 60º.

Use the cosine rule to solve for the resultant force:
(Cosine Rule: a² = b² + c² – 2bc cosA)

