Question

Two charges of `-7 C` and `-4 C` are positioned on a line at points `-9` and `7`, respectively. What is the net force on a charge of `4 C` at `-2`?

Answer 1

5.14x `10^9` Newtons

Explanation:

The charges you have described are very large, so the Forces are also very large. Usually Coulombs are measured in micro (`10^-6`)
or nano (`10^-9`). Anyway, here is what I did, I hope it helps.

Since the charges are in line, we do not have to find the components of any of the vectors.

The questions is about Electric Fields. Every charge creates an electric field. The strength of the field is dependent on the magnitude of the charge. (In this case it is `-4`C and`-7`C). Here is a picture of an electric field:

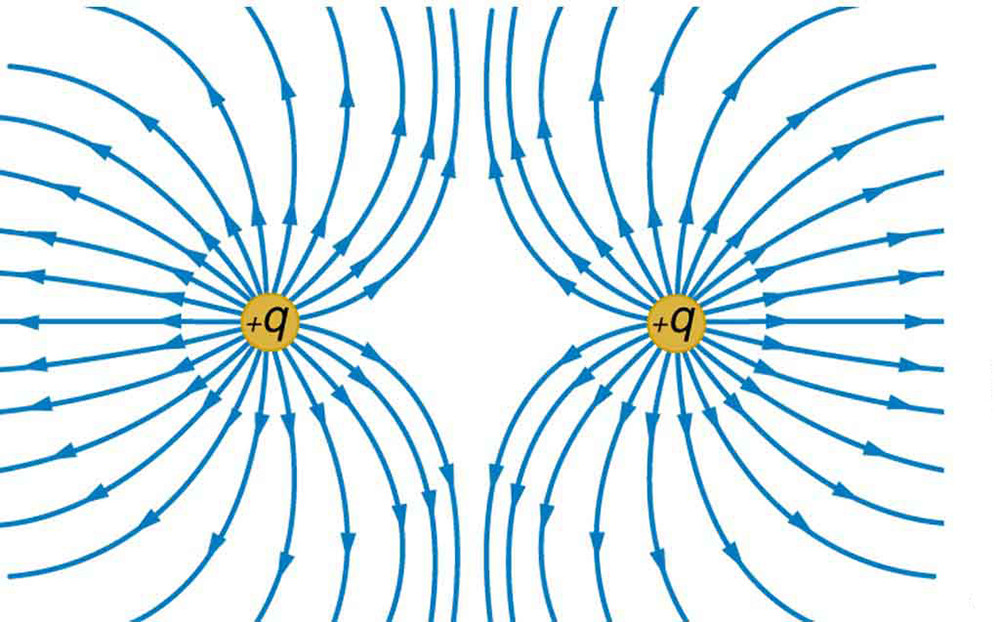
Similarly, the charges from the above question will repel each other, since they are both negative.

Here is the math.

You can calculate the strength of an electric field from the equation: `E=(kq)/r^2` . The "k" is a constant called Coulomb's Constant and it is `8.99 X10^9 Nm^2/C^2`. More can be read about here

To calculate force of a charge, we use the equation `F=qE`.

From here it is a matter of identifying your charges and calculating. For me:
`q_1= -4C`
`q_2= -7C`

The electric field of `q_2` is `E_q= k q_2/r^2`. If we substitute or variables, we get `E_q= 8.99 X10^9 (-4)/7^2`. This is `Eq= 1.28x10^9 N/C`

Now that we have found the Electric Field of `q_2`, we can find the Force on `q_1` by using the `F=qE` equation. When we substitute we get `F= (-4) 1.28x10^9`.

Therefore the force of `q_2` on `q_1` at `-2` is `F= 5.14x10^9 N`.

Here is a good animation and activity: here This animation allow you to see how the force increses as charges are placed nearer or farther away from each other.