How do you find the remaining side of a #30^circ-60^circ-90^circ# triangle if the side opposite #60^circ# is 4?
1 Answer
Explanation:
A
The
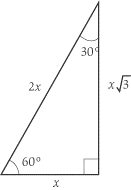
If the side opposite to
#xsqrt 3 = 4#
#x = 4/sqrt3# #-># divide both sides by#sqrt3#
#x = 4/sqrt3 * color(red)(sqrt3/sqrt3)# #-># rationalize the denominator by multiplying by#sqrt3/sqrt3# , which is equal to#1#
#x = (4sqrt3)/3# #-># multiply
Lastly, to find the side opposite to the
#2x = 2 * (4sqrt3)/3 = (8sqrt3)/3#
So, the sides are

