How do you graph #y=2cot4x#?

1 Answer
Gaya3
Oct 7, 2017

#darr darr darr darr#

Explanation:

#1.# Find the period, phase shift, & vertical shift

Using #a *cot(bx+c)+d#,
where:

  • #a=2#
  • #b=4#
  • #c=0#
  • #d=0#

Period #=pi/b=pi/4#... One cycle completes at #pi/4#

Phase shift #=c/b=0/4=0#...Phew! that means no problem

Vertical shift #=d=0#... Again no problem!

#2.# Now graph

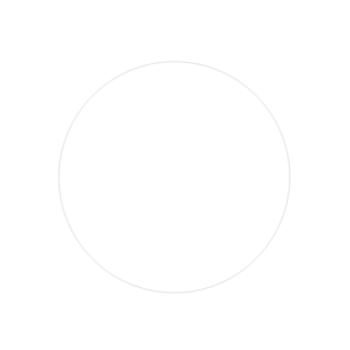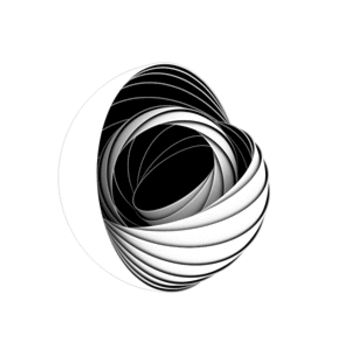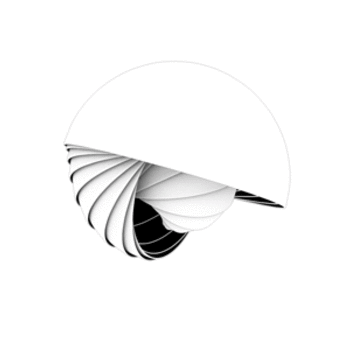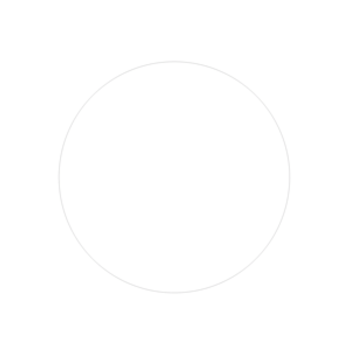
At #pi/8#, (half of the period), #y = 0 #
enter image source here

At #pi/16#, (half of the #pi/8#), #y = a=2 #

At #(3pi)/16#, (batween #pi/8# and the period), #y = -a=-2 #
enter image source here

Three points are #-> (pi/16,2), (pi/8,0), ((3pi)/16,-2)#

Therefore, #y=2cot4x->#
https://www.mathway.com/Precalculus

Related questions
See all questions in Graphing Tangent, Cotangent, Secant, and Cosecant
Impact of this question
5108 views around the world
You can reuse this answer
Creative Commons License