Question

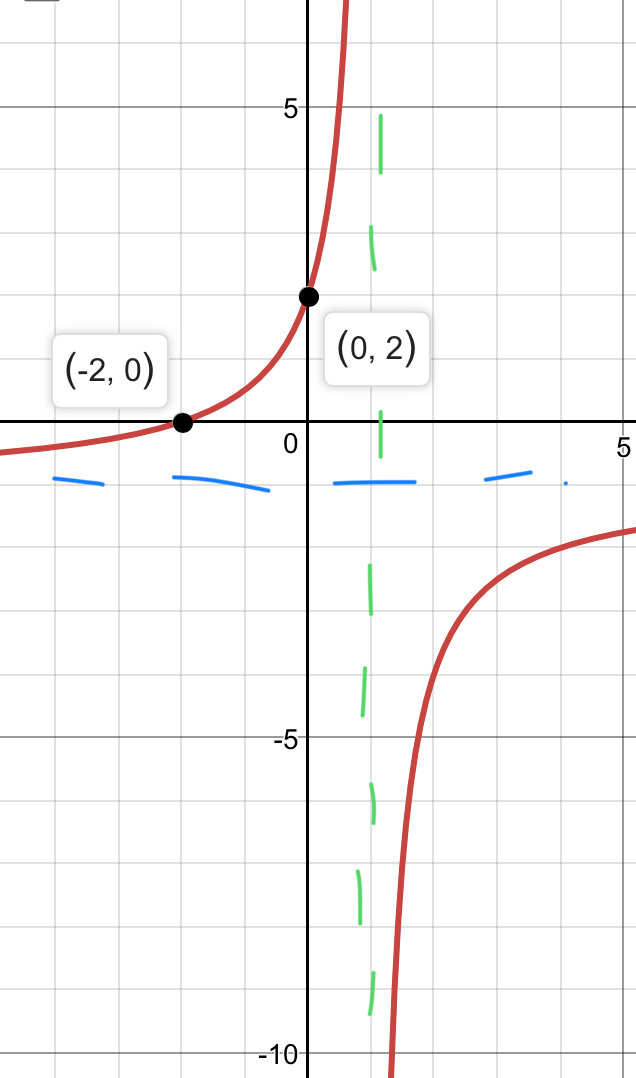
How do you graph `f(x)=-3/(x-1)-1` using holes, vertical and horizontal asymptotes, x and y intercepts?

Answer 1

The green line is the vertical asymptote, the blue line is the horizontal asymptote.

Explanation:

Our first step is to make this function completely rational by combining the two fractions:

`f(x)=-3/(x-1)-1`

`f(x)=-3/(x-1)-(x-1)/(x-1)=(-3-x+1)/(x-1)`

`f(x)=(-2-x)/(x-1)`

There are no holes because the factors do not cancel on the top and bottom.

There are vertical asymptotes anywhere the denominator equals zero so:

`x-1=0->x=1` Thus a vertical asymptote at x=1.

Since the degree is the same on the top and bottom, the horizontal asymptote is determined by dividing the leading coefficients in the numerator and denominator. So `y=-1/1=-1` is a horizontal asymptote.

Find the x-intercept by setting the numerator equal to zero, so `-2-x=0->x=2`

Finally find the y-intercept by setting `x=0`, so:
`f(0)=(-2-(0))/(0-1)=-2/-1=2`.

After plotting these points and graphing these asymptotes just make sure the curve crosses through these points while also slowly approaching the asymptotes as the graph approaches infinity.