If a #1 kg# object moving at #3 m/s# slows down to a halt after moving #8 m#, what is the friction coefficient of the surface that the object was moving over?
2 Answers
Explanation:
It's very useful to organize the given information in a table to be able to identify the formulas that would be efficient to use.

The acceleration is the variable we need to solve for to acquire the net force on the object. The formula,
The acceleration that slows this object down comes from the frictional force from the surface that the object is sliding on which is defined as
( In the following picture, the direction of movement it to the right, the forces interacting on the y-axis, normal force and gravitational force, are of equal magnitude, and the frictional force is opposite to the motion.)
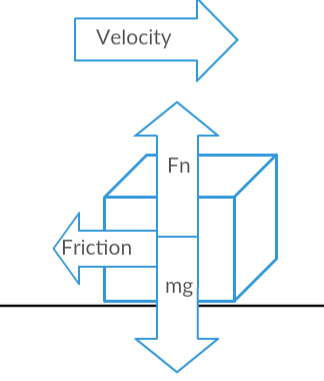
The net force is equal to the frictional force only since the upward normal force cancels out the downward gravitational force therefore,
Where we can then substitute
Explanation:
Well, the force of friction
And the normal is given by...
So now, we need to calculate an acceleration to get the force of friction. We are assuming in this question that


