Question

Which of these ions will have a zero crystal field splitting energy in an octahedral complex?

`(1)" Fe"^(3+)` in a low-spin system
`(2)" Fe"^(3+` in a high-spin system
`(3)" Cr"^(3+)` in a low-spin system
`(4)" Cr"^(3+)` in a high-spin system

Answer 1

`"Fe"^(3+) ->` high spin

Explanation:

Right from the start, you can eliminate options (1) and (3) because those ions form a low spin complex.

Now, I will not go into too much detail about crystal field theory in general because I assume you're familiar with it.

So, you know that transition metal ions placed in symmetric fields have degenerate d-orbitals that are higher in energy than they would have been in an isolated cation.

When you place such a cation in a field with octahedral symmetry, the five degenerate d-orbitals will split into two `e_g` orbitals, which are higher in energy, and three `t_(2g)` orbitals, which are lower in energy.

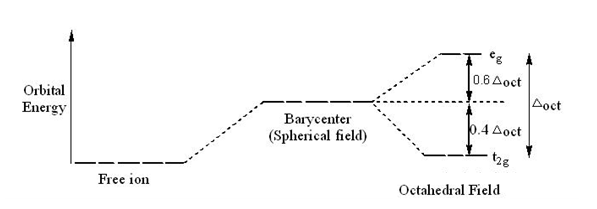
The crystal field stabilization energy, or CFSE, `Delta`, is defined as the stability gained by the ion after placing it in a crystal field.

The idea here is that electrons placed in the `t_(2g)` orbitals will increase the stability of the ion because these orbitals are lower in energy than the degenerate d-orbitals.

On the other hand, electrons placed in the `e_g` orbitals will reduce the stability of the ion because the orbitals are higher in energy than the degenerate d-orbitals.

A low field complex is characterized by the fact that the electrons found in the d-orbitals are all placed in the lower energy `t_(2g)` orbitals. This means that the CFSE for such an ion cannot be equal to zero, since the ion is gaining stability relative to the initial energy level of the d-orbitals.

However, a high spin complex has the potential of having a zero CFSE if the increase in stability resulting from the electrons being placed in the `t_(2g)` orbitals is cancelled out by the decrease in stability resulting from electrons being placed in the `e_g` orbitals.

So, your two candidates are `"Fe"^(3+)` and `"Cr"^(3+)`, which have the following electron configurations

`"Fe"^(3+): ["Ar"] 3d^5 " "` and `" " "Cr"^(3+): ["Ar"] 3d^3`

As you can see, the chromium(III) cation only has three electrons in its d-orbitals, which means that it cannot form a high-spin complex. All three electrons will thus be placed in the lower energy `t_(2g)` orbitals.

Let's do the calculations for the iron(III) cation to make sure that its CFSE will indeed be equal to zero.

So, the iron(III) cation will have three electrons in the lower energy `t_(2g)` orbitals and two electrons in the higher energy `e_(g)` orbitals.

In an octahedral; field, for every electron placed in a `t_(2g)` orbital, you need to add `2/5 * Delta_"oct"` to the total. At the same time, for every electron placed in the `e_g` orbitals, you need to subtract `3/5 * Delta_"oct"` from the total.

This means that you have

`Delta = overbrace( 3 xx 2/5 Delta_"oct")^(color(blue)("3 electrons in " t_(2g))) - overbrace(2 xx 3/5Delta_"oct")^(color(red)("2 electrons in " e_g)) = 0`

The answer will indeed be (2) `"Fe"^(3+) ->` high spin