Question

Question #b7819

Answer 1

`1.8 * 10^(25)`

Explanation:

For starters, you know that aluminium has an atomic number of `13`, which means that a neutral atom of aluminium contains `13` protons inside its nucleus and `13` electrons surrounding the nucleus.

In order to become a `3+` cation, a neutral atom of aluminium must lose `3` electrons. This means that every `"Al"^(3+)` cation contains `10` electrons surrounding its nucleus.


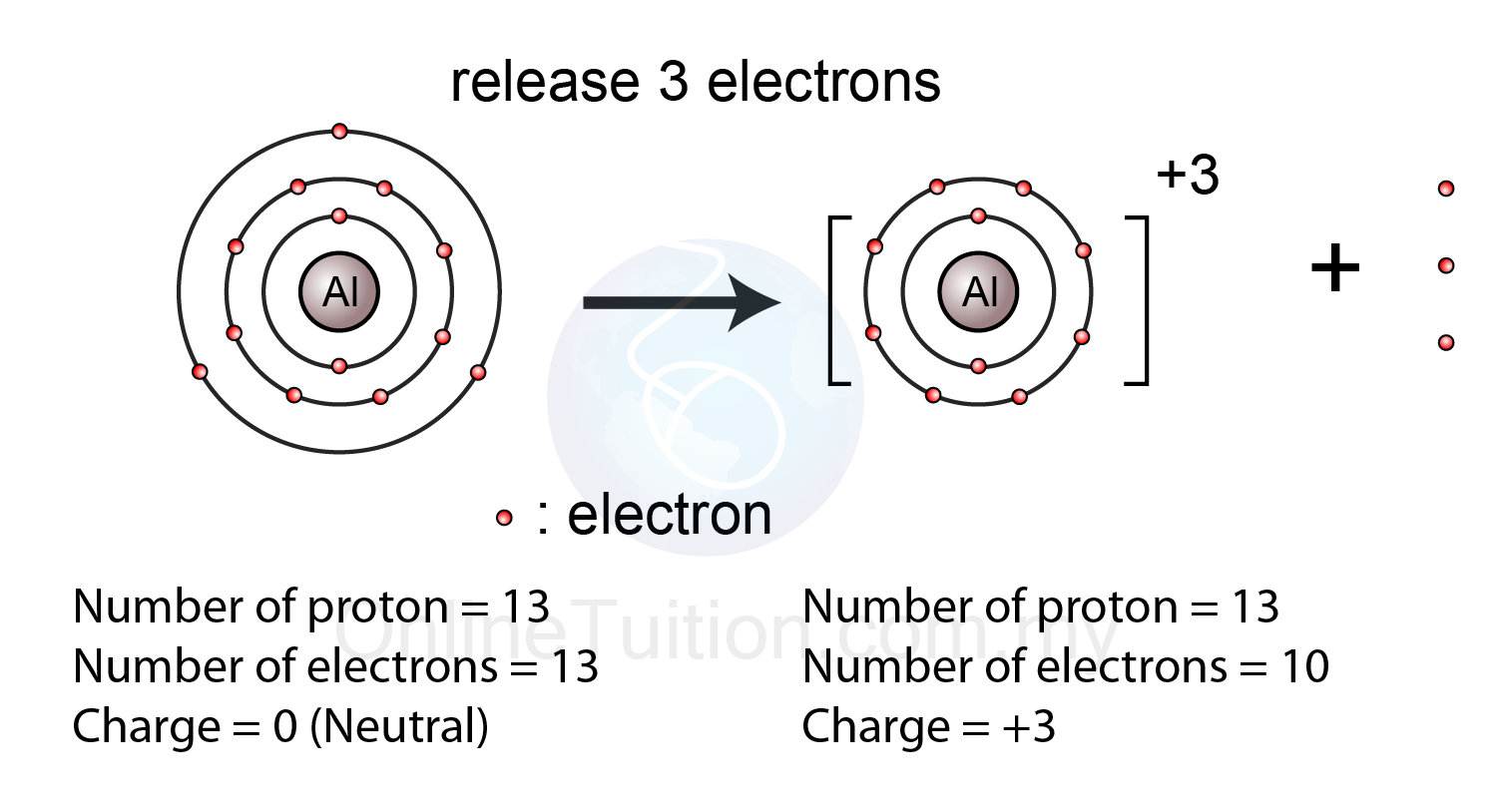
Now, you can safely assume that the mass of an aluminium cation is equal to the mass of a neutral atom of aluminium.

This implies that you can use the molar mass of aluminium to calculate the number of moles of aluminium cations present in your sample

`81 color(red)(cancel(color(black)("g"))) * "1 mole Al"^(3+)/(27.0color(red)(cancel(color(black)("g")))) = "3.0 moles Al"^(3+)`

As you know, in order to be able to say that you have `1` mole of aluminium cations, you need to have `6.022 * 10^(23)` aluminium cations in your sample.

This means that your sample will contain

`3.0 color(red)(cancel(color(black)("moles Al"^(3+)))) * overbrace((6.022 * 10^(23)color(white)(.)"Al"^(3+)color(white)(.)"cations")/(1color(red)(cancel(color(black)("mole Al"^(3+))))))^(color(blue)("Avogaro's constant")) = 1.81 * 10^(24)` `"Al"^(3+)` `"cations"`

Finally, use the fact that every aluminium cation contains `10` electrons to say that your sample contains

`1.81 * 10^(24)color(red)(cancel(color(black)("Al"^(3+)"cations"))) * "10 e"^(-)/(1color(red)(cancel(color(black)("Al"^(3+)"cations")))) = color(darkgreen)(ul(color(black)(1.8 * 10^(25)color(white)(.)"e"^(-))))`

The answer is rounded to two sig figs, the number of sig figs you have for the mass of `"Al"^(3+)`.