Question

A balanced lever has two weights on it, the first with mass `42 kg` and the second with mass `14 kg`. If the first weight is `2 m` from the fulcrum, how far is the second weight from the fulcrum?

Answer 1

`color(blue)(x= 6m)`

Did you know you can treat units of measurement the same way as numbers? I have shown this in my solution.

Explanation:

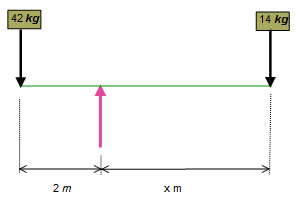
For the system to be in equilibrium (not rotating or moving in any way) all the 'moments' have to cancel each other out.

`color(blue)("Taking moment about the fulcrum.")`

`color(brown)("Consider the LHS of the fulcrum")`

`42 kg xx 2m = 84 kgm -> "force "xx" distance")`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(brown)("Consider the RHS of the fulcrum")`

`14 kg xx xm=14x kgm`
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Equating LHS to RHS for equilibrium gives:

`42 kg xx 2m = 14 kg xx xm`

`=> 84 kgm = 14x kgm`

Remember that the units for `x` is m

Divide both sides by `14 kg` (leaves `x m` on the RHS)

`=> 84/14 (kgm)/(kg)= xcolor(white)(.)m`

`6 color(white)(.)(cancel(kg)m)/(cancel(kg)) =xcolor(white)(.)m`

So `x= 6m`