Question

A projectile is shot from the ground at a velocity of `23 m/s` and at an angle of `(3pi)/4`. How long will it take for the projectile to land?

Answer 1

The total flight time of the projectile is `~~3.3s`

Explanation:

Assuming the launch and landing points are at equal altitudes, we can use a kinematic equation to determine the flight time from the launch of the projectile to its maximum altitude (`v_f=0`) and multiply by two to get the total flight time.

`v_f=v_i+a_yDeltat`

`=> Deltat=(v_f-v_i)/a_y`

`=>Deltat=(-v_i)/a_y`

We know that when an object is in free fall, the acceleration is equal to `-9.8m/s^2` (vertically).

Because the projectile is launched at an angle, we will need to break the velocity up into components. This can be done using basic trigonometry.

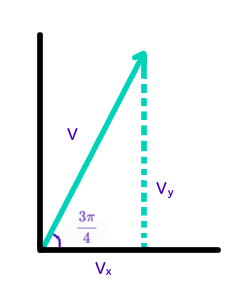
Where `v` is the initial velocity, and `v_x` and `v_y` are the horizontal and vertical components of the velocity, respectively. We can see that:

`v_x=vcos(theta)`
`v_y=vsin(theta)`

We will only require the `y` component.

`v_y=23*sin((3pi)/4)= (23sqrt(2))/2m/s`

We can now calculate the rise time of the projectile.

`Deltat=(-v_i)/a_y`

`Deltat=(-(23sqrt(2))/2m/s)/(-9.8m/s^2)`

`Deltat=~~1.66s`

The total flight time is then `~~3.3s`