Question

A projectile is shot from the ground at an angle of `pi/6` and a speed of `9 m/s`. Factoring in both horizontal and vertical movement, what will the projectile's distance from the starting point be when it reaches its maximum height?

Answer 1

`~~4.04m`

Explanation:

The velocity of projection of the projectile `u=9ms^-1`
The angle of projection of the projectile `alpha=pi/6`
The horizontal component of velocity of projection
`u_h=ucosalpha=9xxcos(pi/6)=4.5sqrt3ms^-1`
TheVertical component of velocity of projection
`u_v=ucosalpha=9xxsin(pi/6)=4.5ms^-1`

````````````````````````````````````````````````````````````````````````````````````````````````````
Assuming the ideal situation where gravitational pull is the only force acting on the body and no air resistance exists , we can easily proceed for various calculation using equation of motion under gravity.

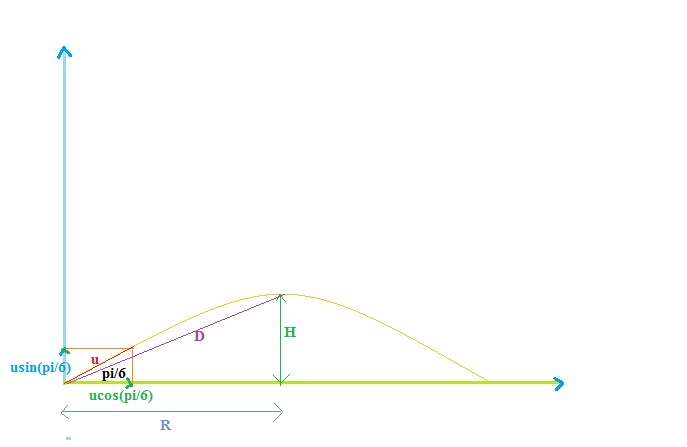
CALCULATION
Let the projectile reaches its maximum height H m after t s of its start.
The final vertical component of its velocity at maximum height will be zero
So we can write
`0 = usinalpha-gxxt =>t = (usinalpha)/g=4.5/10=0.45s` taking `g=10ms^-2`

Again
`0^2=(usinalpha)^2-2xxgxxH`
`=>H = (usinalpha)^2/g=(4.5)^2/10=2.025m`

During `t=1.25s` of its ascent its horizontal component will remain unaltered and that is why the horizontal displacement
`R = ucosalpha xxt=4.5sqrt3xx0.45m=3.5m`

Hence the projectil's distance ( D ) from starting point to the maximum point of its ascent is given by
`D=sqrt(R^2+H^2)=sqrt(3.5^2+(12.025)^2)~~4.04m`