Question

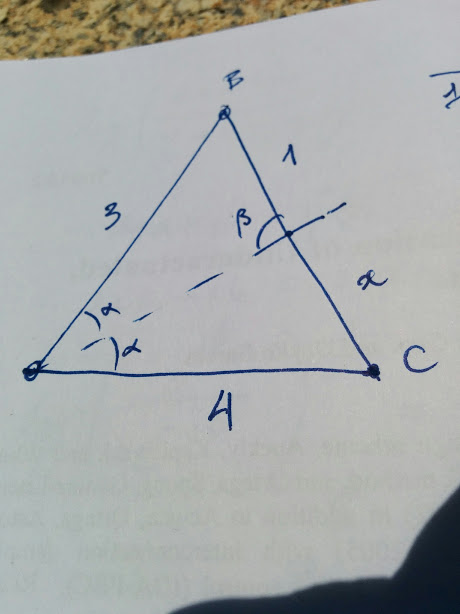
A triangle has corners points A, B, and C. Side AB has a length of `3`. The distance between the intersection of point A's angle bisector with side BC and point B is `1`. If side AC has a length of `4`, what is the length of side BC?

Answer 1

`7/3`

Explanation:

Given

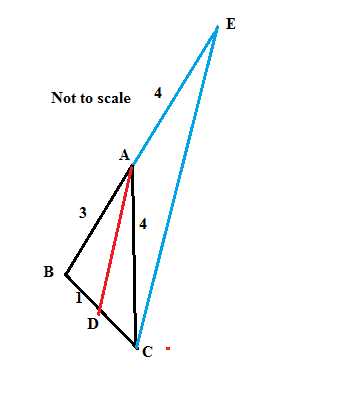
• `In Delta ABC, AB =3,AC=4`
• `AD",the bisector of " /_BAC,"intersects BC at D" and BD=1`
• We are to find out the length of BC

Construction

• `CE" is drawn parallel to DA, it intersects produced BA at E"`

Now

• `DA||CE,AC -"transversal"-> /_ DAC="alternate"/_ ACE`

• `DA||CE,BE-"transversal"-> /_ BAD="corresponding"/_ AEC`

• `But /_BAD =/_DAC,"AD being bisector of " /_BAC`

• `:.In" "DeltaACE,/_ACE=/_AEC=>AC=AE=4`

• `" Now "DA||CE->"BD"/"DC"="BA"/"AE"=3/4`

• `"BD"/"DC"=3/4=>1/"DC"=3/4=>DC=4/3`

• `BC=BD +DC=1+4/3=7/3`

Answer 2

`bar(BC)=7/3`

Explanation:

According to the figure attached, using sinus law we have

`1/sin(alpha)=3/sin(beta)`
`x/sin(alpha)=4/sin(pi-beta)=4/sin(beta)`

so we have

# { (sin(beta)=3 sin(alpha)), (x sin(beta) =4 sin(alpha)) :} #

Then we obtain `x = 4/3` and `bar(BC)=1+x=7/3`