Question

An isosceles triangle has sides A, B, and C, such that sides A and B have the same length. Side C has a length of `24` and the triangle has an area of `48`. What are the lengths of sides A and B?

Answer 1

`A = B = 4sqrt(10)`

Explanation:

Just to clear up what the last contributor had to say, I will write a second answer.

Here is a diagram of the given problem.

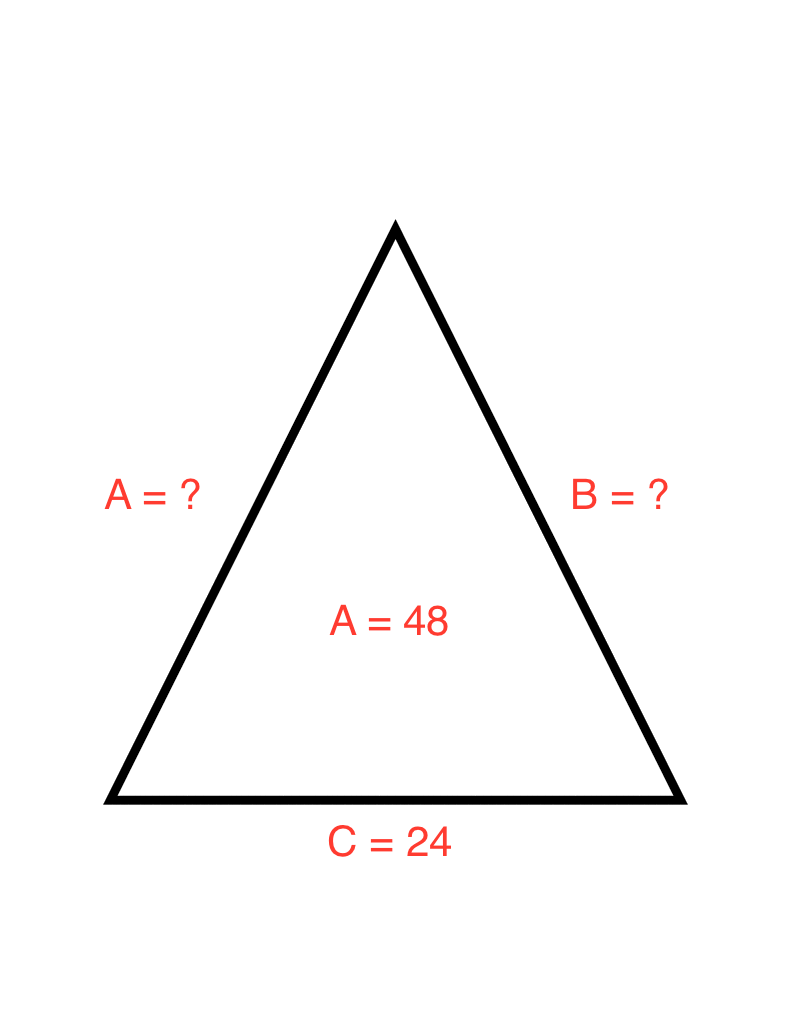
You must understand for this problem is that the formula for area of a triangle is `A = (b xx h)/2`. We already know the base and the area, so it's logical that we will have to find the height before finding `A` and `B`.

`48 = (24 xx h)/2`

`96 = 24h`

`h = 4`

Here's an updated diagram with the height included.

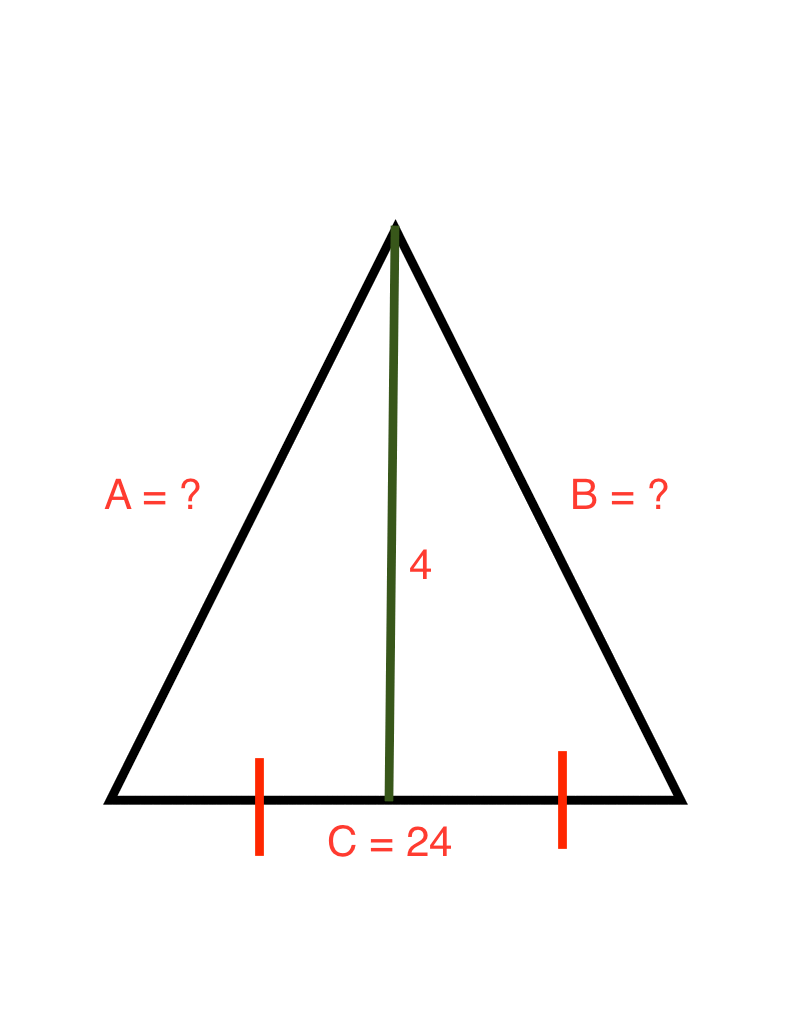
As you can see, I've added the two red bars to signify that the base is cut into two congruent lengths by the height, since we're dealing with an isosceles triangle.

Since the entire base measures `24`, half of the base measures `12`. Now, we are now left with two right triangles, as shows the following diagram.

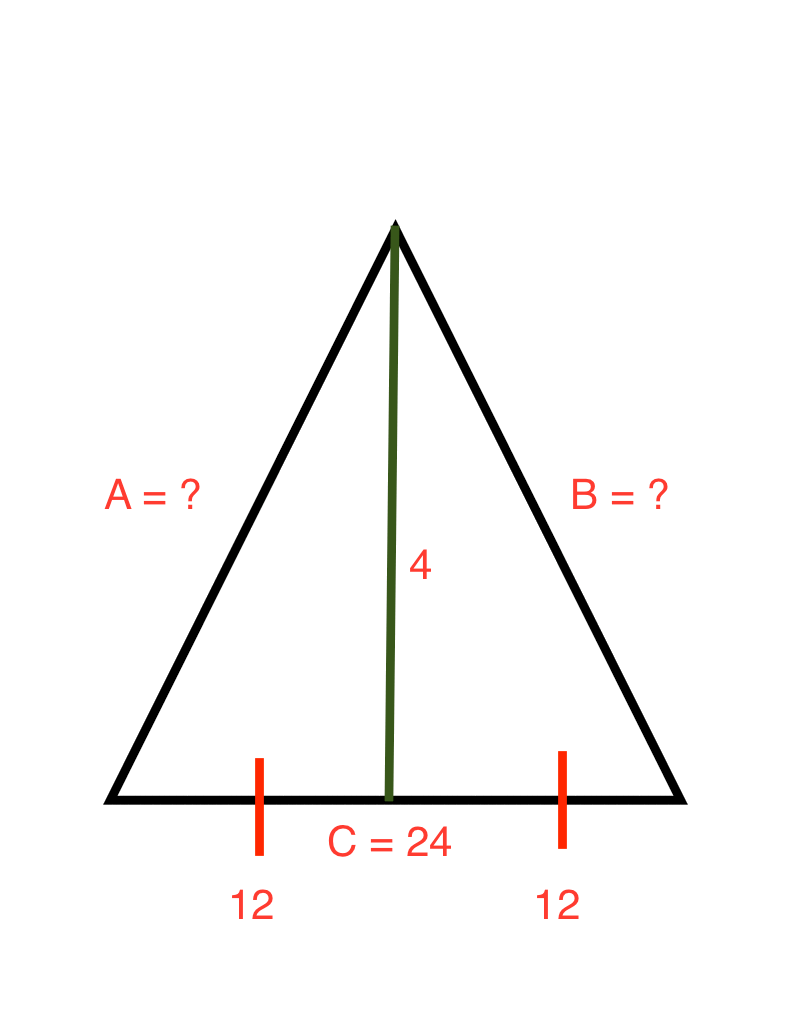
Using pythagorean theorem, we can state:

`12^2 + 4^2 = A^2`

`144 + 16= A^2`

`sqrt(160) = A`

`A = 4sqrt(10)`