Question

An object with a mass of `24 kg` is hanging from an axle with a radius of `16 m`. If the wheel attached to the axle has a radius of `48 m`, how much force must be applied to the wheel to keep the object from falling?

Answer 1

The force is `=78.4N`

Explanation:

The load is `L=24gN`

The radius of the axle is `r=16m`

The radius of the wheel is `R=48m`

The effort is `=FN`

The acceleration due to gravity is `g=9.8ms^-2`

Taking moments about the center of the axle

`F*48=24g*16`

`F=(24g*16)/48=78.4N`

The force is `F=78.4N`

Answer 2

`78.4052N` to 4 decimal places

Explanation:

`color(blue)("Preamble")`

Did you know that units of measurement can be manipulated in the same way that numbers can. If you are ever not sure what to do with the numbers look at what you have to do to the units to give you your target.

`color(white)()`

The mass of 24 kg converted to downward force in Newtons is:

`24cancel(kg)xx9.80065 N/(cancel(kg)) = 235.2156N` to 4 decimal places

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("Answering the question")`

Presenting opposing forces about the shaft centre line of the shaft as if it is a fulcrum we have:

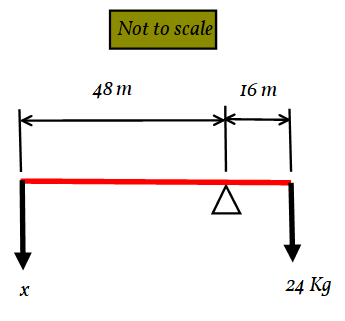
If this condition is not 'in equilibrium' (all balanced out) then the system would be in motion.

Taking moments about the fulcrum

`48x_1Kgm=16xx24 Kgm`

But force is measure in Newtons by changing the units of measurement we have:

`48x_2Nm=16xx235.2156 Nm`

Divide both sides by `48m` (gets `x` Newtons on its own)

`(48m)/(48m)xx x_2N=(16xx235.2156)/48 color(white)("d")(Ncancel(m))/cancel(m)`

But `(48m)/(48m) = 1 and 1xx xN=xN`

`x_2N=78.4052N` to 4 decimal places