Question

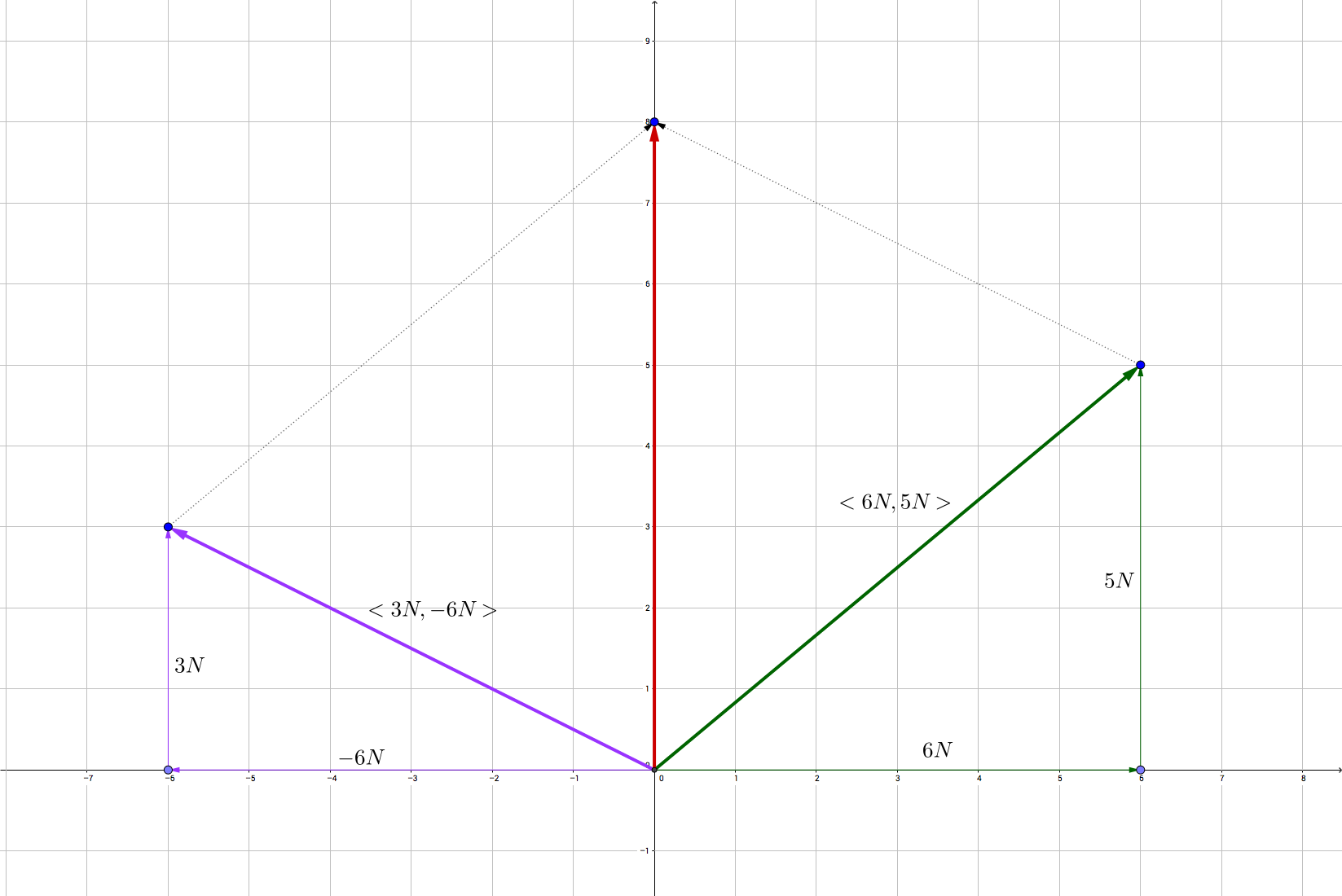
An object with a mass of `4 kg` is acted on by two forces. The first is `F_1= < -6 N , 3 N>` and the second is `F_2 = < 6 N, 5 N>`. What is the object's rate and direction of acceleration?

Answer 1

The acceleration is `2` `m/s^2` and the angle is `90^\circ`.

Explanation:

When you want to sum two vectors in classical mechanics, it is enough if you sum them component by component.

The final vector is

`F=F_1+F_2=<-6N+6N, 3N+5N>`

`=<0N, 8N>`

The length of a vector is given by

`L=sqrt(x^2+y^2)`

that of our vector is

`L=sqrt(0^2+8^2)=8`.

Then the force is with a magnitude of 8N and the acceleration is given by

`F=ma`

`a=F/m=8/4=2` `m/s^2`

The direction is given by

`\theta=arctan(y/x)=arctan(8/0)`

This of course is a problem because we cannot divide by zero. On the other hand we know that the arctan is singular when the angle is `pi/2`. So the direction is an angle of `pi/2` or `90^\circ`.