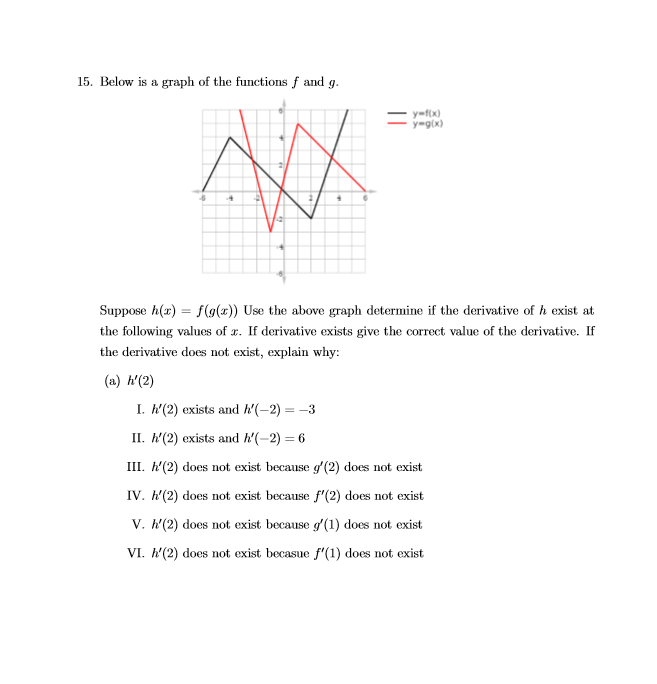
Find #h'(2)#? (see image below)

enter image source here

1 Answer
Truong-Son N.
Mar 14, 2017

The graphs above were:

Some things to keep in mind:

  • Note that #h(x) = f(g(x))#. This means you will have to find a value for #g(x)#, and use that value for the argument of #f(x)#. For instance, for some #g(3) = w#, we have that #h(3) = f(g(3)) = f(w)#.
  • We should recognize that the derivative at a corner, e.g. when the graph abruptly changes slope, is undefined.
  • #h'(x) = f'(g(x))*g'(x)# by the chain rule.

Thus, #h'(x)# does not exist if #g(x)# or #g'(x)# do not exist. (If #f'(x)# does not exist at some specified point, it doesn't necessarily imply that #g(x)# does not exist.)

Reading from the above graph:

For #h'(2)#, we have that:

#h'(2) = f'(g(2))g'(2)#

#= f'(4)g'(2)#

#= 3*-1#

#= -3#

Therefore, #h'(2) = -3#, and it exists. Two of the question options apparently has us check #h'(-2)#, so let's see.

#h'(-2) = f'(g(-2))g'(-2)#

#= f'(1)g'(-2)#

#= -1*-4#

#= 4#

Well, we found that #h'(2)# exists... but neither of the answer choices in which it exists has the correct #h'(-2)#. Either there is a typo in the question or there is no correct answer choice.

Related questions
See all questions in Understanding the Gradient function
Impact of this question
8085 views around the world
You can reuse this answer
Creative Commons License