Help finding gravitational attraction and speed?
Several planets (Jupiter, Saturn, Uranus) are encircled by rings, perhaps composed of material that failed to form a satellite. In addition, many galaxies contain ring-like structures. Consider a homogeneous thin ring of mass 3.0 x 10^22 kg and outer radius 6.8 x 10^8 m (the figure). (a) What gravitational attraction does it exert on a particle of mass 86 kg located on the ring's central axis a distance 4.0 x 10^8 m from the ring center? (b) Suppose that, starting at that point, the particle falls from rest as a result of the attraction of the ring of matter. What is the speed with which it passes through the center of the ring?
Several planets (Jupiter, Saturn, Uranus) are encircled by rings, perhaps composed of material that failed to form a satellite. In addition, many galaxies contain ring-like structures. Consider a homogeneous thin ring of mass 3.0 x 10^22 kg and outer radius 6.8 x 10^8 m (the figure). (a) What gravitational attraction does it exert on a particle of mass 86 kg located on the ring's central axis a distance 4.0 x 10^8 m from the ring center? (b) Suppose that, starting at that point, the particle falls from rest as a result of the attraction of the ring of matter. What is the speed with which it passes through the center of the ring?
1 Answer
We will be using Newton's Law for Gravitation:
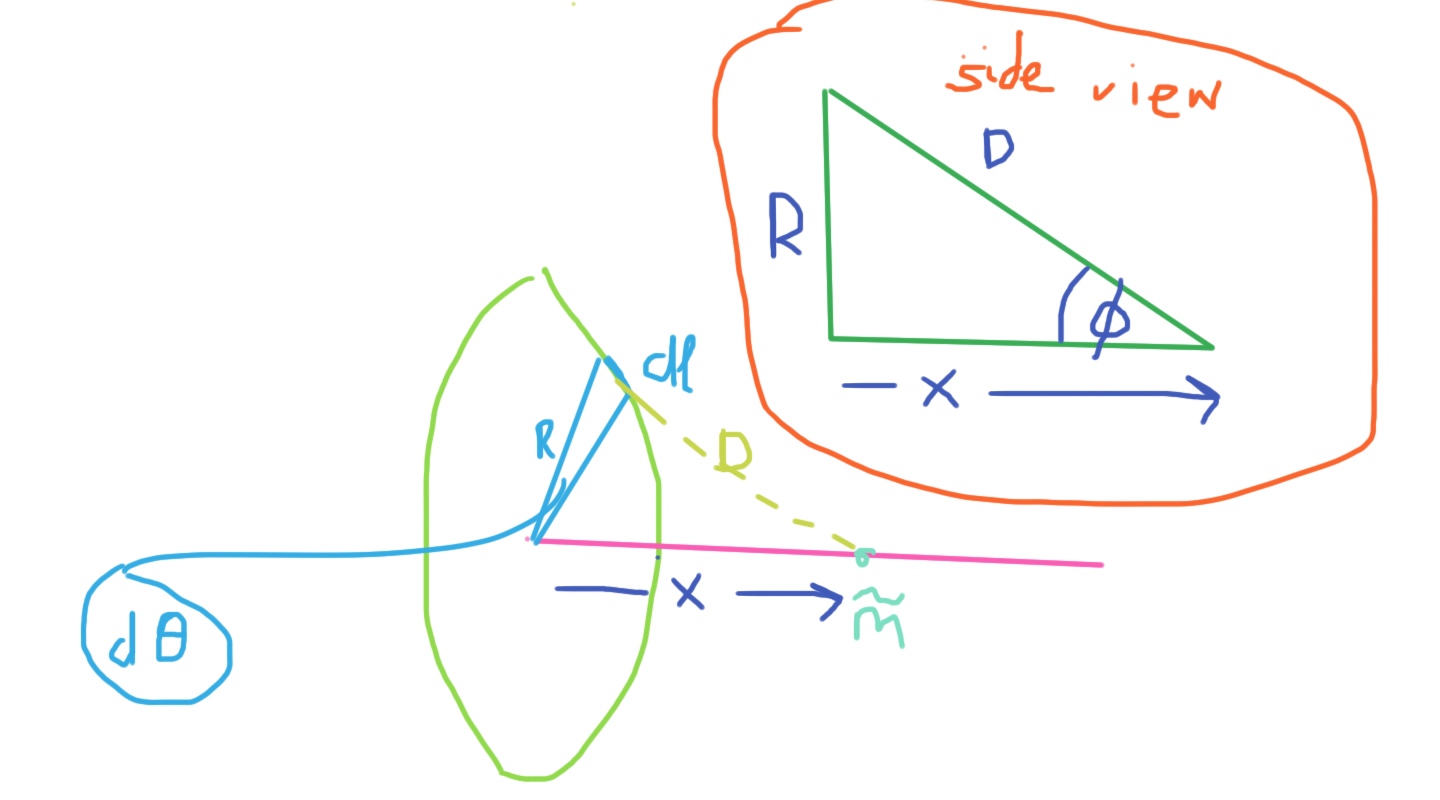
The green ring in the drawing radius
So, for the small arc,
The particle of mass
The side view shows that from symmetry the distance
So from Newton's Law:
That is the magnitude of the force between the particle and the small element in the ring. Again we see symmetry demands that the particle be pulled along the axis of the ring. We need to resolve
Putting it all together:
Part (a)
Part (b)
There are several ways to do this. We can calculate the potential energy
In a conservative system, that energy becomes kinetic energy for the particle:

