How do you convert #r^2 = 9cos5(theta)# into cartesian form?

Question

2927 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-12-07T09:36:50

#1/9 (x^2+y^2)^3.5-x^5+10x^3y^2-5xy^4=0#. Graph is inserted

Here, #9 cos 5theta = r^2 >=0#. So, #5theta# is in Q1 or Q4.

And so, #theta# is in #2kpi+-(1/5)pi/2#, k = 0, 1, 2, 3...

The period for the graph is #(2pi)/5=72^o#.

For one half (#36^o#) #r^2# is positive. For the other, #r^2< 0#.

Only for half period = #pi/5 = 36^o#, the graph appears.

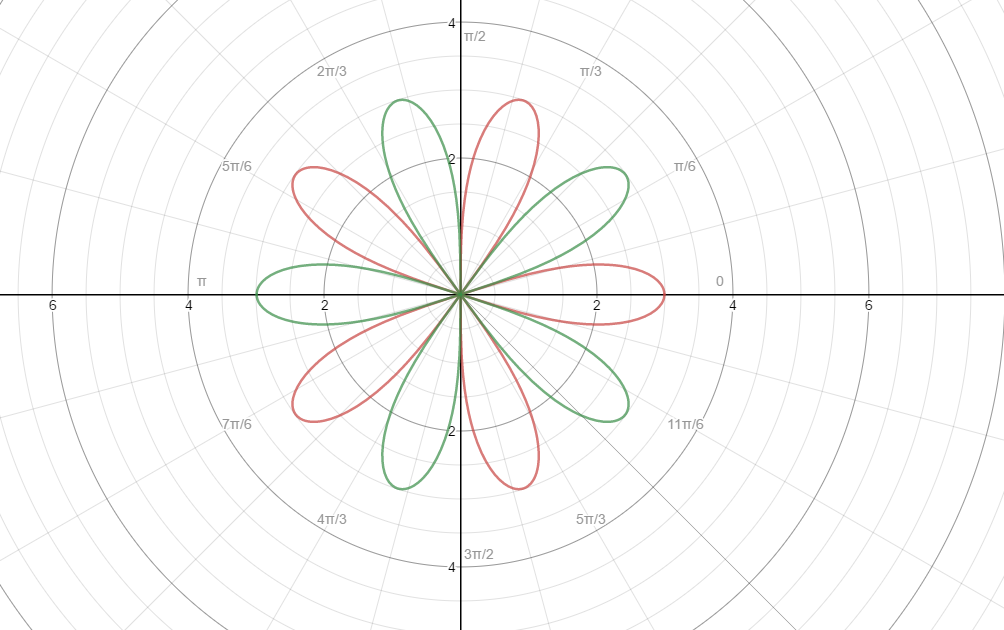
I expect five equal loops like the ones that are marked red in the

second graph, contributed by the other author.

The conversion formula is #r(cos theta, sin theta)=(x, y)#, with

#r=sqrt(x^2+y^2)>=0#.

Substitution gives the form in the answer.

graph{(x^2+y^2)^3.5-9x^5+90x^3y^2-45xy^4=0}

Debugging was elusive for me, for so long. I was able to do it now.

Adikesavan's revised answer ends here.

Here is a graph where the red is the positive value for r and the green is the negative values for r:
Desmos.com