Question

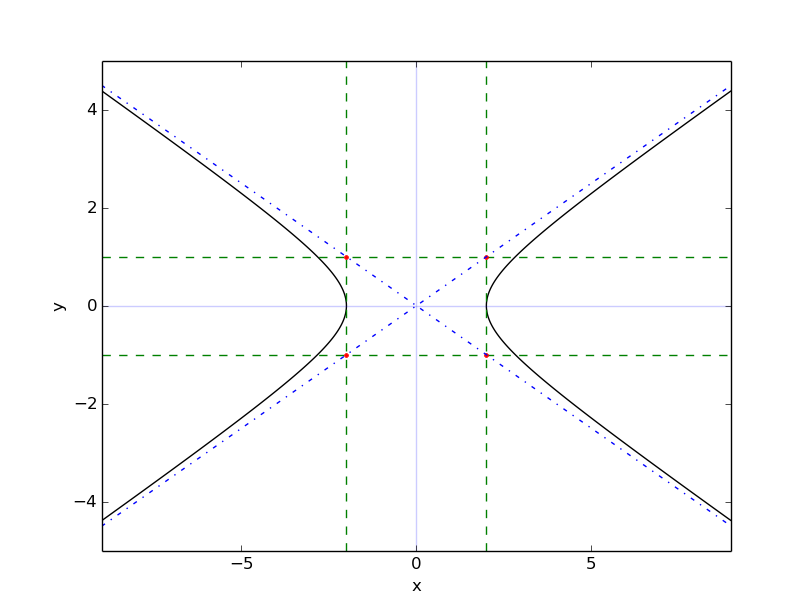
How do you find the center and vertices and sketch the hyperbola `y^2/1-x^2/4=1`?

Answer 1

Write your equation in form: `(y-y_c)^2/a^2 -(x-x_c)^2/b^2=1`
where the center is `C=(x_c,y_c)`

Explanation:

Your equation is already in the right form.
You just read it:
Center: `C=(0,0)`, `a=1`, `b=2`
Draw a rectangle `2b` width and `2a` length.
[Alternative: draw `x=-2` , `x=2`, `y=1`, `y=-1` (green lines)]
through opposite rectangle's vertices [or intersection of green lines] (red points) draw a line- this 2 lines are hyperbola asymptotes.

So you know hyperbola have the vertices at `(0,-2)` and `(0,2)` and is "going towards" the asymtotes.

Asiptote is a line such that the distance between the curve and the line approaches zero as one or both of the x or y coordinates tends to infinity