Question

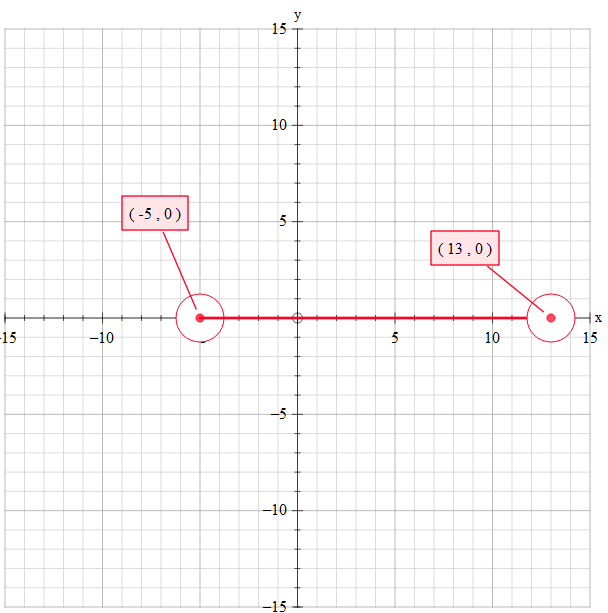
How do you graph and solve `|x-4|<9`?

Answer 1

`-5 < x < 13`

Explanation:

When working with absolute value inequalities, we need to remember that the absolute value function will return a positive value regardless of what lies within. For instance, if we have

`x=3`, `x` can be 3 or it can be `-3`. And so in our question we need to address this:

`abs(x-4)<9=>x-4 < pm9=> -9 < x-4 < 9`

We can now solve this by adding 4 to all sides:

`-9 < x-4 < 9`

`-9 color(red)(+4)< x-4color(red)(+4) < 9color(red)(+4)`

`-5 < x < 13`

We can graph this on a number line by placing hollow circles around `-5` and 13 (to indicate that all the points up to but not including `-5` and 13 are part of the solution) and then drawing a line connecting the two.