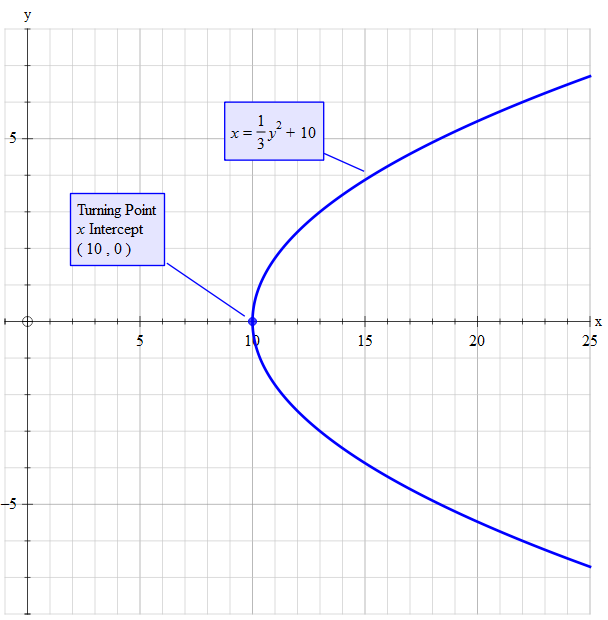
How do you graph the parabola #x = 1/3y^2 + 10# using vertex, intercepts and additional points?

Question

1789 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-03-15T23:30:03

The axis of symmetry is the x-axis

x-intercept is at #(x,y)=(10,0)#

This is a quadratic in #y# instead of in #x#

The #1/3y^2# term is positive so the graph is of general shape #sub#

Consider the general form of: #x=at^2+by+c#

#b=0# so the axis of symmetry is the x-axis

At #y=0; x=10# so the x-intercept is at #(x,y)=(10,0)#

There is no y-intercept as the shape is #sub# and #x_("intercept")=10#

Tony B