How do you solve #2(2+3)+4x=2(2x+2)+6#?
1 Answer
All real numbers or
Explanation:
First, simplify
Put it back into the equation:
Next, use the distributive property to simplify
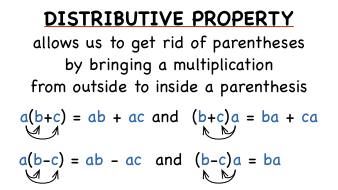
Following this image, we know that:
Put it back into the equation:
Add
Subtract
Oh no! Our variables are gone now. Now we see if this equation is true. It is true that
Hope this helps!

