How do you solve #(n-9)/(n+5)=7/4#?
↳Redirected from
"Question #f98b4"
See a solution process below:
First, cross multiply the equation:
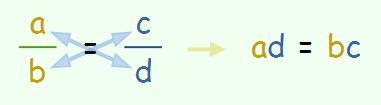
#(color(orange)(n) - color(orange)(9))/(color(orange)(n) + color(orange)(5)) = color(blue)(7)/color(blue)(4)#
#color(blue)(4)(color(orange)(n) - color(orange)(9)) = color(blue)(7)(color(orange)(n) + color(orange)(5))#
#(color(blue)(4) * color(orange)(n)) - (color(blue)(4) * color(orange)(9)) = (color(blue)(7) * color(orange)(n)) + (color(blue)(7) * color(orange)(5))#
#4n - 36 = 7n + 35#
Next, subtract #color(red)(4n)# and #color(blue)(35)# from each side of the equation to isolate the #n# term while keeping the equation balanced:
#-color(red)(4n) + 4n - 36 - color(blue)(35) = -color(red)(4n) + 7n + 35 -
color(blue)(35)#
#0 - 71 = (-color(red)(4) + 7)n + 0#
#-71 = 3n#
Now, divide each side of the equation by #color(red)(3)# to solve for #n# while keeping the equation balanced:
#-71/color(red)(3) = (3n)/color(red)(3)#
#-71/3 = (color(red)(cancel(color(black)(3)))n)/cancel(color(red)(3))#
#-71/3 = n#
#n = -71/3#
In this equation there is one fraction on each side. The easiest method is to cross multiply. (See below for why it works).
#(n-9)/(n+5) = 7/4#
#7(n+5) = 4(n-9)#
#7n +35 = 4n -36#
#7n -4n = -36-35#
#3n = -71#
#n = -71/3#
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
To get rid of the denominators, multiply both sides by the LCM of the denominators, which is #4(n+5)#
#(4(n+5))/1 xx (n-9)/(n+5) = (4(n+5))/1 xx 7/4" "larr# cancel
#(4cancel((n+5)))/1 xx (n-9)/cancel((n+5)) = (cancel4(n+5))/1 xx 7/cancel4#
This leaves you with: #4(n-9) = 7(n+5)#
Which is exactly the result from cross-multiplying.


