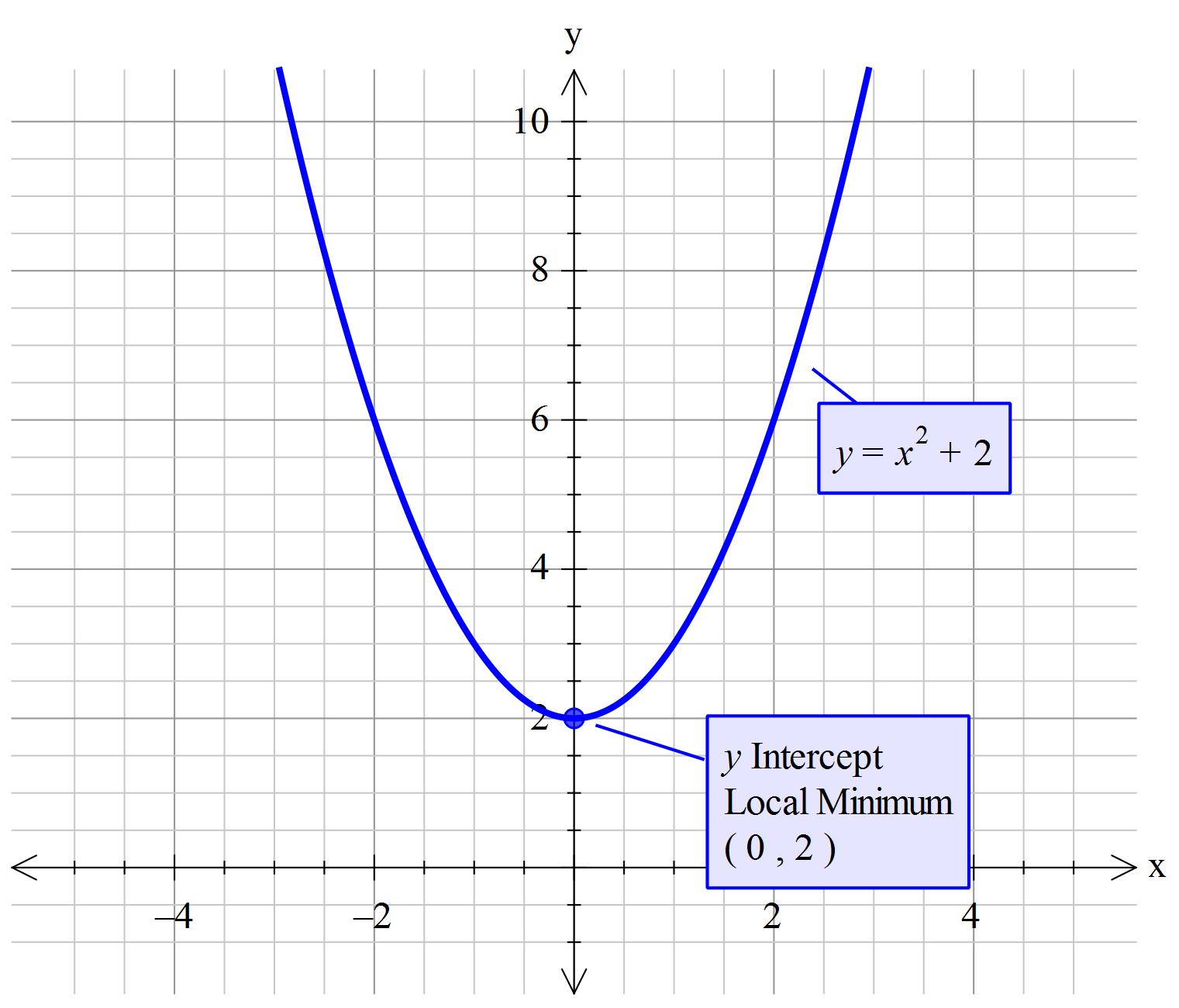
How do you use the important points to sketch the graph of #y=x^2+2#?
1 Answer
See explanantion
Explanation:
Determining the key points improves the precision for your sketch. It also demonstrates that you have some grasp of what the equation is doing.
Standard form;
The coefficient of
There is not any
The constant lifts or lowers the whole graph depending on its sign
As we have a constant of + 2 it lifts the whole graph up by 2
As the graph is of form
it has no x-axis intercept.
The y-intercept is at
y-intercept = 2
As you only have one key point (y-intercept) I would suggest that you do a quick determination of two other points to guide your hand drawn sketch. For example: