How many solutions do the system of equations #2x-3y=4# and #4x-6y =-7# have?

1 Answer
Gió
Feb 5, 2015

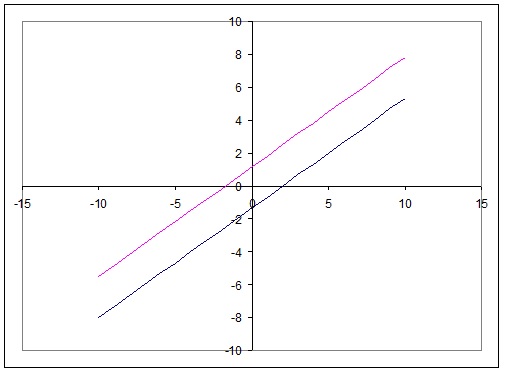
Each equation in your system represents a straight line...the only thing is that the two lines in your case are parallel!!!! So your system doesn't have solutions (basically the two lines never meet!!!).
You can see this because the second equation has the #x# and #y# coefficients equals to those of the first but multiplied by #2#!!!
Basically it means that your two lines have the same slope (inclination) and cross the #y# axis in diferent places:
Rearranging you get:
#2x-3y=4# that can be written as:
#y=2/3x-4/3#

#4x-6y=-7# that can be written as:
#y=4/6x+7/6=2/3x+7/6#

You can see that the slope of your two lines (the coeficient of #x#) is the same, i.e., #2/3# the only difference is the #y#-axis crossings: #-4/3# and #7/6#.
Graphically you can see this even more easily plotting the two lines as:
enter image source here

Related questions
See all questions in Consistent and Inconsistent Linear Systems
Impact of this question
8621 views around the world
You can reuse this answer
Creative Commons License