Question

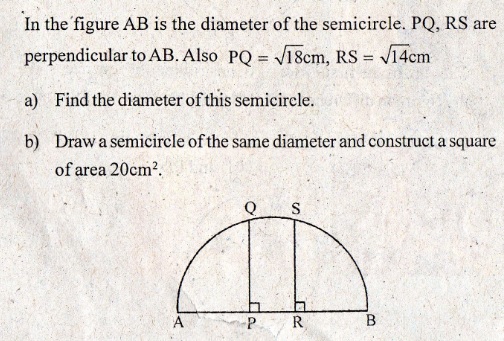
In the figure AB is the diameter of the circle. PQ, RS are perpendicular to AB. Also `"PQ" = sqrt(18) "cm"`, `"RS" = sqrt(14) "cm"`. Find the diameter of this semicircle? Draw a semicircle of the same diameter and construct a square of area `20 "cm"^2`?

Answer 1

drawn
Trying to get a solution considering positive integer values of lengths of `AP,PB,AR,RB`

(a) In the given figure `AB` is the diameter of a semicircle, `PQ,RS` are perpendicular to `AB`

`PQ=sqrt18`

As `PQ` is mean proportion of `AP and PB` we get

`sqrt(APxxPB)=sqrt(3xx6)`

Again
`RS=sqrt14=sqrt(7xx2)`

As `RS` is mean proportion of `AR and RB` we get

`sqrt(ARxxRB)=sqrt(7xx2)`

So above two relations give a possible length of diameter of the semicircle.

`AB=3+6=7+2=9`cm

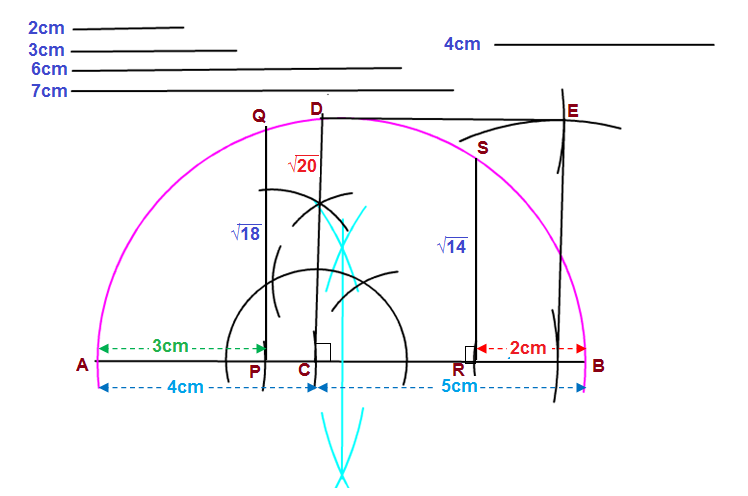
(b) A semicircle of diameter `AB=9cm` is drawn. `AC=4cm` is cut off from `AB`.

The length of remaining part will be `CB=(9-4)cm=5cm`.

A perpendicular `CD` is drawn on `AB` at `C`, which intersects the semicircle at `D`. It will be mean proportion of `AC and CB`
Hence `CD^2=ACxxCB=4xx5cm^2=20cm^2`
.
The square `BCDE` drawn on `CD` will have area `=20cm^2`