Question

Show that the area of a triangle is `A_Delta = 1/2 bxxh` where b is the base and h the altitude of the traingle?

Answer 1

Please see below.

Explanation:

While considering the area of a triangle there are three possibilities.

1. One base angle is right angle, other will be acute.
2. Both base angles are acute, and lastly
3. One base angle is obtuse, other will be acute.

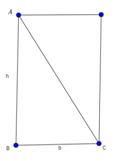
1 Let the triangle be right angled at `B` as shown and let us complete the rectangle, by drawing perpendicular at `C` and drawing a parallel line from `A` as below. Now area of rectangle is `bxxh` and hence area of triangle will be half of it i.e.`1/2bxxh`.

2 If the triangle has both acute angles at base, draw perpendiculars from `B` and `C` and also from `A` downwards. Also a draw a line parallel to `BC` from `A` cutting perpendiculars from `B` and `C` at `D` and `E` respectively as shown below.

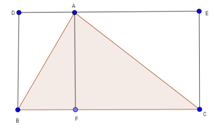
Now, as area of triangle `ABF` is half of rectangle `ADBF` and area of triangle `ACF` is half of rectangle `AECF`. Adding the two, area of triangle `ABC` is half of rectangle `DBCE`. But as area of latter is `bxxh`, area of triangle will be half of it i.e.`1/2bxxh`.
3 If the triangle has one obtuse angle at the base say at `B`, draw perpendiculars from `B` and `C` upwards and also from `A` downwards meeting extended `CB` at `F`. Also a draw a line parallel to `BC` from `A` cutting perpendiculars from `B` and `C` at `D` and `E` respectively as shown below.

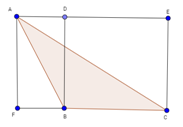
Now, as area of triangle `ABF` is half of rectangle `ADBF` and area of triangle `ACF` is half of rectangle `AECF`. Subtracting the area of triangle `ABF` from triangle `ACF` and also of rectangle `ADBF` from rectangle `AECF`, we get that area of triamgle `ABC` is half of rectangle `DBCE`. But as area of latter is `bxxh`, area of triangle will be half of it i.e.`1/2bxxh`.