Question

Suppose a circle of radius r is inscribed in a hexagon. What is the area of the hexagon?

Answer 1

Area of a regular hexagon with a radius of inscribed circle `r` is
`S=2sqrt(3)r^2`

Explanation:

Obviously, a regular hexagon can be considered as consisting of six equilateral triangles with one common vertex at the center of an inscribed circle.

The altitude of each of these triangles equals to `r`.

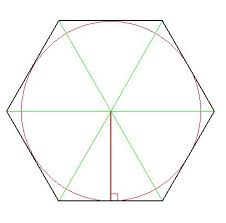
The base of each of these triangles (a side of a hexagon that is perpendicular to an altitude-radius) equals to
`r*2/sqrt(3)`

Therefore, an area of one such triangle equals to
`(1/2)*(r*2/sqrt(3))*r=r^2/sqrt(3)`

The area of an entire hexagon is six times greater:
`S = (6r^2)/sqrt(3) = 2sqrt(3)r^2`