Question

The area of a square is 45 more than the perimeter. How do you find the length of the side?

Answer 1

Length of one side is 9 units.
Rather than doing a straight factorising approach I have used the formula to demonstrate its use.

Explanation:

As it is a square the length of all the sides is the same.
Let the length of 1 side be L
Let the area be A

Then `A=L^2`............................(1)

Perimeter is `4L`........................(2)

The question states: "The area of a square is 45 more than.."

`=> A=4L+45`.................................(3)

Substitute equation (3) into equation (1) giving:

`A=4L+45=L^2....................(1_a)`

So now we are able to write just 1 equation with 1 unknown, which is solvable.
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

`4L+45=L^2`

Subtract `L^2` from both sides giving a quadratic.

`-L^2+4L+45=0`
The conditions that satisfy this equation equalling zero gives us the potential size of L

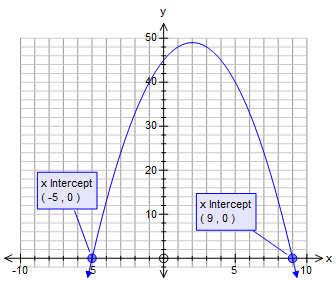
Using `ax+bx+c=0` where `x= (-b+-sqrt(b^2-4ac))/(2a)`

`a=-1`
`b=4`
`c=45`

`x=(-4+-sqrt((4)^2-4(-1)(45)))/(2(-1))`

`x=(-4+-14)/(-2)`

`x= (-18)/(-2) = +9`

`x=(+10)/(-2)=-5`

Of these two `x=-5` is not a logical length of side so

`x=L=9`

`"Check "-> A= 9^2= 81 "units"^2`

`4L= 36 -> 81-36= 45`

So area does indeed equal sum of sides + 45