The equations #{(y = c x^2+d, (c > 0, d < 0)),(x = a y^2+ b, (a > 0, b < 0)):}# have four intersection points. Prove that those four points are contained in one same circle ?

Question

2214 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-09-14T14:35:18

See below.

Making the following transformations

#{(y=cx^2+d),(x=ay^2+b):} ->{(y/c = x^2+d/c),(x/a=y^2+b/a):}->x^2+y^2-x/a-y/c+d/c+b/a=0#

and comparing with the generic circle equation

#C->(x-x_0)^2+(y-y_0)^2-r_0^2=0#

#{(x^2+y^2-x/a-y/c+d/c+b/a=0),(x^2+y^2-2x_0x-2y_0y+x_0^2+y_0^2-r_0^2=0):}#

we conclude that with #(c > 0, d < 0, a > 0, b < 0)#

#{(x_0=1/(2a)),(y_0=1/(2c)),(r_0=sqrt(1/(2a)^2+1/(2c)^2-(d/c+b/a))):}#

#C# represents a circle which contains the solutions.

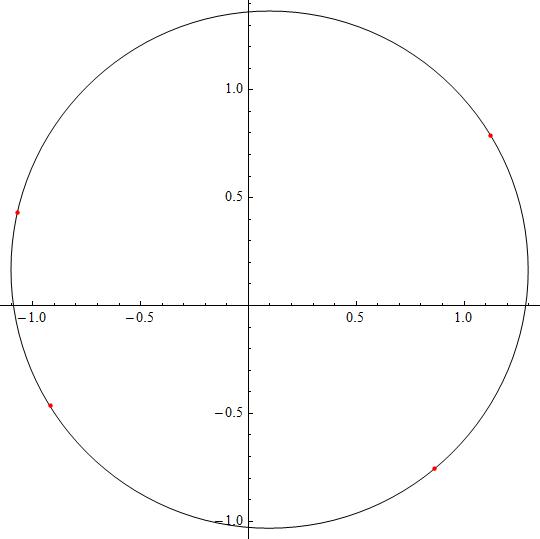
As an example, using the values

#a = 5, b = -2,c = 3,d = -3#

we obtained the following outcome.

enter image source here