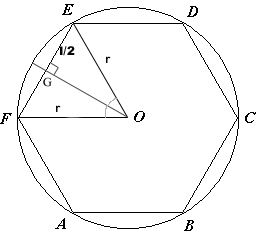
The perimeter of a regular hexagon is 48 inches. What is the number of square inches in the positive difference between the areas of the circumscribed and the inscribed circles of the hexagon? Express your answer in terms of pi.

Question

5151 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-08-05T02:15:19

#color(blue)("Diff. in area between Circumscribed and Inscribed circles "#

#color(green)(A_d = pi R^2 - pi r^2 = 36 pi - 27 pi = 9pi " sq inch"#

Perimeter of regular hexagon #P = 48 "inch"#

Side of hexagon #a = P / 6 = 48 / 6 = 6 " inch"#

Regular hexagon consists of 6 equilateral triangles of side a each.

Inscribed circle : Radius #r = a / (2 tan theta), theta = 60/2 = 30^@ #

#r = 6 / (2 tan (30)) = 6 / (2 (1/sqrt3)) = 3 sqrt 3 " inch"#

#"Area of inscribed circle " A_r = pi r^2 = pi (3 sqrt3)^2 = 27 pi " sq inch"#

#"Radius of circumscribed circle " R = a = 6 " inch"#

#"Area of circumscribed circle "A_R = pi R^2 = pi 6^2 = 36 pi " sq inch"#

#"Diff. in area between Circumscribed and Inscribed circles "#

#A_d = pi R^2 - pi r^2 = 36 pi - 27 pi = 9pi " sq inch"#