Question

The radio waves of a particular AM radio station vibrate 680,000 times per second. What is the wavelength of the wave?

Answer 1

`"440 m"`

Explanation:

Frequency and wavelength have an inverse relationship described by the equation

`color(blue)(lamda * nu = c)" "`, where

`lamda` - the wavelength of the wave
`nu` - its frequency
`c` - the speed of light in a vacuum, usually given as `3 * 10^8"m s"^(-1)`

So, what does this inverse relationship tell you?

Well, it tells you that waves that have longer wavelengths will have lower frequencies, and waves that have shorter wavelengths will have higher frequencies.

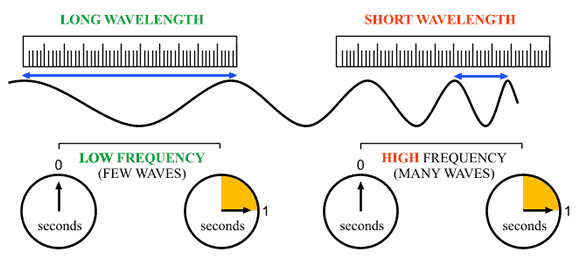
Now, what does it means for something to vibrate `"680,000"` times per second?

As you know, frequency is defined as the number of repeating events per unit of time.

`color(blue)(nu = "events"/"unit of time")`

That unit of time could be one second, one minute, one year, etc.

In your case, you are told that the wave vibrates `"680,000` times per second, so your unit of time will be `"1 s"`. The frequency of the wave will thus be

`nu = "680,000 vibrations"/"1 s" = "680,000 s"^(-1)`

You can write this in scientific notation if you want

`nu = 6.8 * 10^5"s"^(-1)`

This means that the wavelength associated with this wave will be

`lamda * nu = c implies lamda = c/(nu)`

`lamda = (3 * 10^8"m" color(red)(cancel(color(black)("s"^(-1)))))/(6.8 * 10^5color(red)(cancel(color(black)("s"^(-1))))) = 4.412 * 10^(2)"m"`

Rounded to two sig figs, the answer will be

`lamda = color(green)("440 m")`