Question

What is an ellipse?

Answer 1

Image source: (http://www.qrg.northwestern.edu/projects/vss/docs/space-environment/2-how-ellipse-is-different.html)

Explanation:

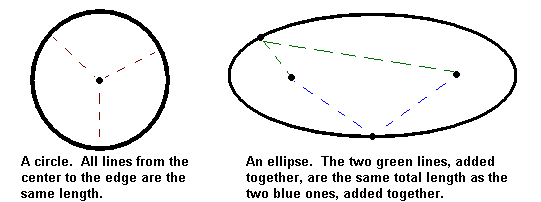
Ellipse Definition: On a plane, ellipse is defined as follows - If two special points (called the foci) are picked on a plane and if we collect all the points around those foci such that the sum of the distances between any point in that collection and the two foci are a constant, then the locus of all these points form a curve called Ellipse.

Though this definition is for ellipse as a plane curve, this definition can be extended to define ellipse on non-planar surfaces, like for example on Earth.

Ellipses are symmetric about exactly two axes that are perpendicular to each other. If we align those two axes along the two cartesian axes `X` and `Y` and have the point of intersection coincide with the coordinate origin then the ellipse can be described by the following simple equation,

Cartesian Equation of an Ellipse: `\frac{x^2}{a^2}+\frac{y^2}{b^2}=1`.

Here `a` is called the semi-major axis and `b` is called the semi-minor axis.

Ellipses are characterised by a parameter called eccentricity (`e`) which is related to the semi-major and semi-minor axes as follows,
`e=\sqrt{1-\frac{b^2}{a^2}}`.

A circle is a special ellipse with eccentricity zero (`e=0`).

If one of the focus is placed at the coordinate origin and measure the angle ( `\theta` ) from the semi-major axis in the counter-clockwise direction, the ellipse of eccentricity `e`, can be described by the following simple polar equation,

`r(\theta) = \frac{a(1-e^2)}{1+e\cos\theta}`