Question

What is the angle between electron groups in the tetrahedral geometry?

Answer 1

This can be geometrically worked out, but we expect an angle of `109.5^@`. Let's verify that by drawing a tetrahedron in a cube.

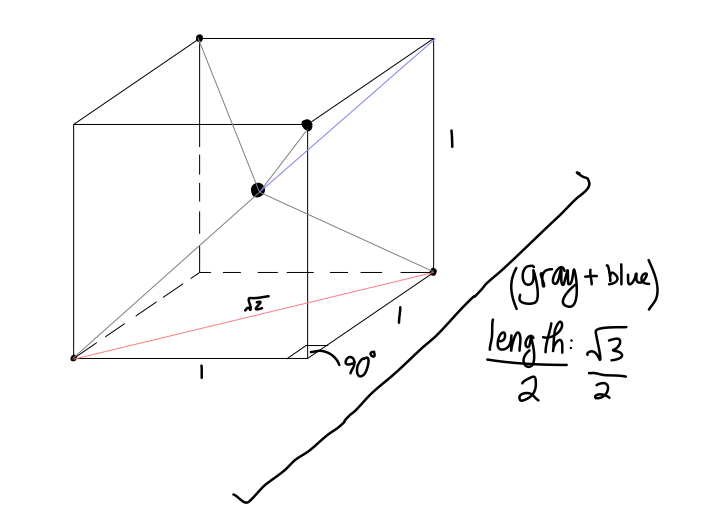
Here,

• the center of the cube is the central atom in a tetrahedral molecule.
• the upper left rear, upper right front, bottom left front, and bottom right rear points are the surrounding atoms in a tetrahedral molecule.

The base diagonal is `sqrt2` from the geometry of a `45-45-90` triangle of leg lengths `1`. From the diagram, the diagonal from the bottom left front to the upper right rear is from the Pythagorean Theorem:

`1^2 + (sqrt2)^2 = c^2`

`=> c = sqrt3`

We want half that diagonal, as that is the length towards the central atom in a tetrahedral molecule, so `c/2 = sqrt3/2`.

Finally, we have an `SSS` triangle to solve to determine the tetrahedral angle. By symmetry, the side lengths are:

• upper-left: `x = sqrt3/2`
• upper-right: `y = sqrt3/2`
• base diagonal: `z = sqrt2`

The law of cosines is:

`z^2 = x^2 + y^2 - 2xycosZ`

We can use this to find the tetrahedral angle, `Z`, corresponding to base diagonal length `z = sqrt2`:

`(z^2 - x^2 - y^2)/(-2xy) = cosZ`

`=> color(blue)(Z) = arccos((z^2 - x^2 - y^2)/(-2xy))`

`= arccos(((sqrt2)^2 - (sqrt3/2)^2 - (sqrt3/2)^2)/(-2(sqrt3/2)(sqrt3/2)))`

`= arccos((2 - 3/4 - 3/4)/(-3/2))`

`= color(blue)(109.5^@)`