Question

What is the area of an equilateral triangle with a side length of 1?

Answer 1

`sqrt3/4`

Explanation:

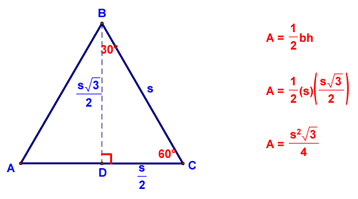
Imagine the equilateral being cut in half by an altitude. This way, there are two right triangles which have the angle pattern `30˚-60˚-90˚`. This means the sides are in a ratio of `1:sqrt3:2`.

If the altitude is drawn in, the base of the triangle is bisected, leaving two congruent segments with length `1/2`. The side opposite the `60˚` angle, the height of the triangle, is just `sqrt3` times the existing side of `1/2`, so its length is `sqrt3/2`.

This is all we need to know, since the area of a triangle is `A=1/2bh`.

We know the base is `1` and the height is `sqrt3/2`, so the area of the triangle is `sqrt3/4`.

Refer to this picture if you're still confused: