Question

What is the dot product of two vectors that are parallel?

Answer 1

It is simply the product of the modules of the two vectors (with positive or negative sign depending upon the relative orientation of the vectors).
A typical example of this situation is when you evaluate the WORK done by a force `vecF` during a displacement `vecs`.
For example, if you have:

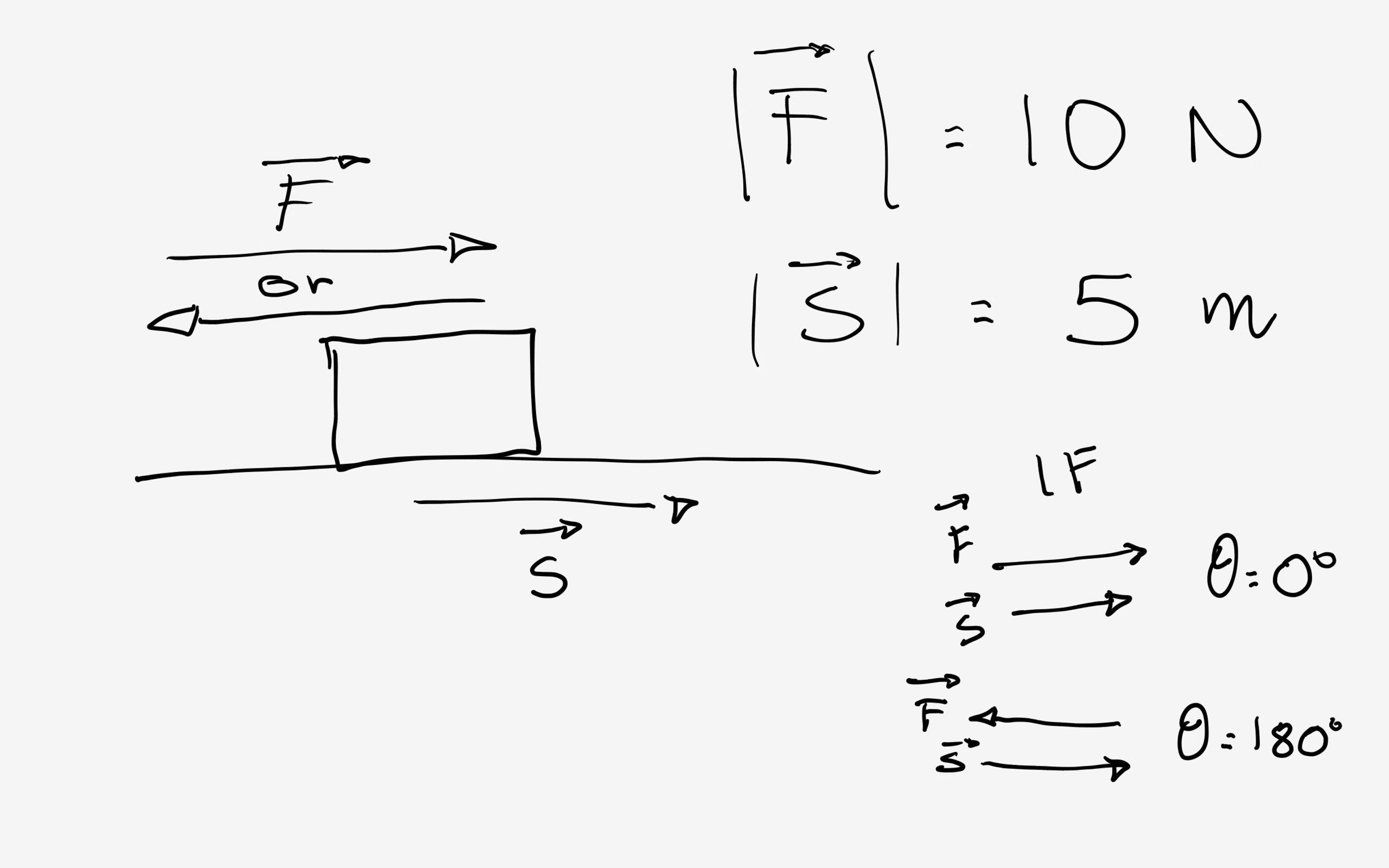
Work done by force `vecF`:
`W=|vecF|*|vecs|*cos(theta)`
Where `theta` is the angle between force and displacement; the two vectors being parallel can give:

`theta=0°` and `cos(theta)=cos(0°)=1` so:
`W=5*10*1=50 J`

Or:

`theta=180°` and `cos(theta)=cos(180°)=-1` so:
`W=5*10*-1=-50 J`