Question

What is the slope, m of the line which goes through the points (a,5) and (3,b)?

Answer 1

`m = (b-5)/(3 - a)`

Explanation:

The slope of a line essentially tells you how the value of `y` changes as you change the value of `x`.

In other words, if you start from a point that lies on a line, the slope of the line helps you find other points that lie on the line.

Now, you already know that `(a,5)` and `(3,b)` are two points that lie on the given line. This means that in order to find the slope, you must figure out how to get from point `(a,5)` to point `(3,b)`.

Let's start with the `x` coordinates. If you start at `x=a` and stop at `x=3`, the change in `x`, or `Deltax`, will be

`Deltax = 3 - a`

Do the same for the `y` coordinates. If you start at `y=5` and stop at `y=b`, the change in `y`, or `Deltay`, will be

`Deltay = b - 5`

Since you know that

`"slope" = m = (Deltay)/(Deltax)`

you can say that you have

`m = (b-5)/(3 - a)`

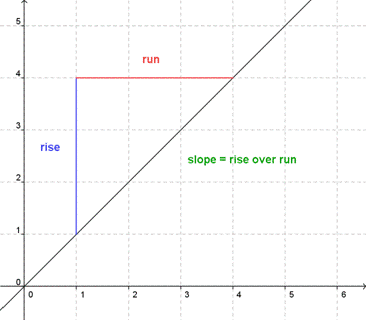
That is the slope of the line. In other words, if you start at any point that is on your line, you can find another point that lies on the line by moving `(3-a)` positions on the `x` axis, i.e. `(3-a)` positions across, or run, and `(b-5)` positions on the `y` axis, i.e. `(b-5)` positions up, or rise.

That is why the slope of the line is said to be rise over run.