Question

What is the relationship among amplitude, crest, and trough?

Answer 1

The relationship is `A =|C-T|/2`, where A is the amplitude value, C is the crest value and T is the value of the trough.

Explanation:

In general, a wave can be defined as the periodic change of one (dependent variable), y, with another (independent variable) x.

In physics, the dependent variable y may be a physical quantity like pressure, voltage, height and the independent variable may be a physical quantity like time, space or something related (but doesn’t have to be).

The amplitude is the difference between the equilibrium value (of the dependent variable) and the maximum value the dependent quantity will take over one period.

Let’s take a concrete example `y=3*sin(x) or y=A*sin(x)`, where `A=3` shown in the graph below. Here, y is the dependent quantity (or variable) and x is the independent quantity. Equilibrium is at zero (i.e. `y(0)=3*sin(0)=0`)

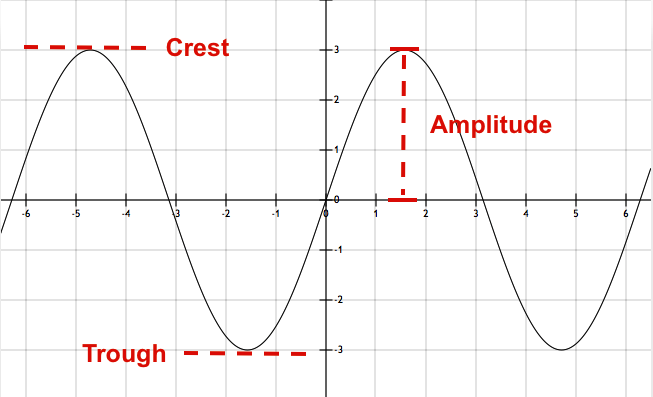
Since the crest of the wave is defined as the highest value (e.g. `y=3`) and the trough is the lowest value (i.e. `y=-3`), The amplitude of a wave, A, is `1/2` the distance between the crest (C=3) and trough (T=-3) of that wave, which we can express in compact form as

`A =(C-T)/2 = [3-(-3)]/2=6/2=3`

So, in this case, `A=3`, half the distance between the crest and the trough of the wave.