Question

How do you factor `4x^2+17x-15`?

Answer 1

Use the slide and divide method to get `(4x-3)(x+5)`.

Explanation:

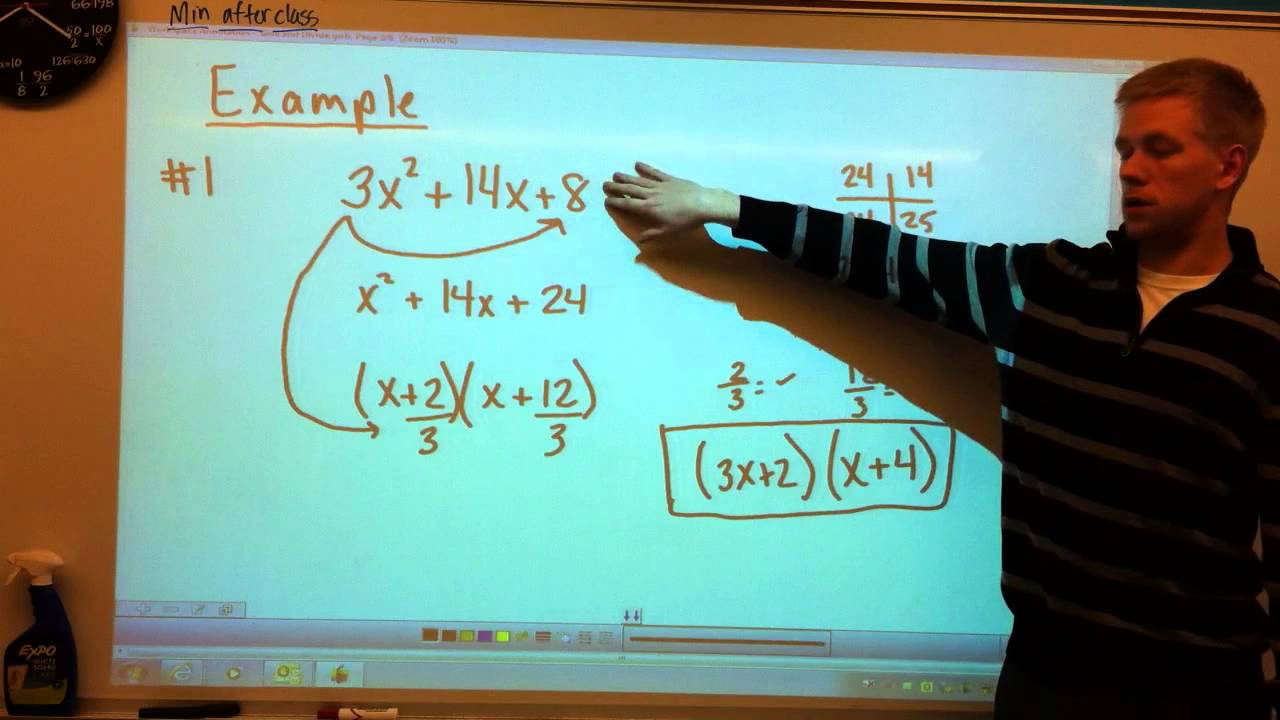
We'd use the slide and divide method.

Start by taking the `a` value, `4`, and multiply it (also called sliding) with the `c` value, `-15`. Now we're working with the equation `x^2+17x-60`.

Then we'd factor it as normal, to get `(x+20)(x-3)`. We have to bring the four back, and divide it from the `20` or the `-3`.

We'd get `(x+(20)/4)` and `(x-(3)/4)`. We only have to divide from one binomial though, and the `20` is easiest to work with.

We'd then have `20/4=5`, so we'd end up with (x+5).

We'd multiply the last `4`, from the second binomial, over to `x`, and get `4x-3`.

Combine the two to get `(4x-3)(x+5)`.