Question

Question #3a151

Answer 1

Use `sintheta` where `theta` is the angle of the ladder.
`DeltaBC=4(sqrt(3)-sqrt(2))`

Explanation:

Alright. First we draw a diagram. I have labelled the points of the triangle `A`, `B`, and `C`. I will also be referring to the angle `/_BAC` as `theta`

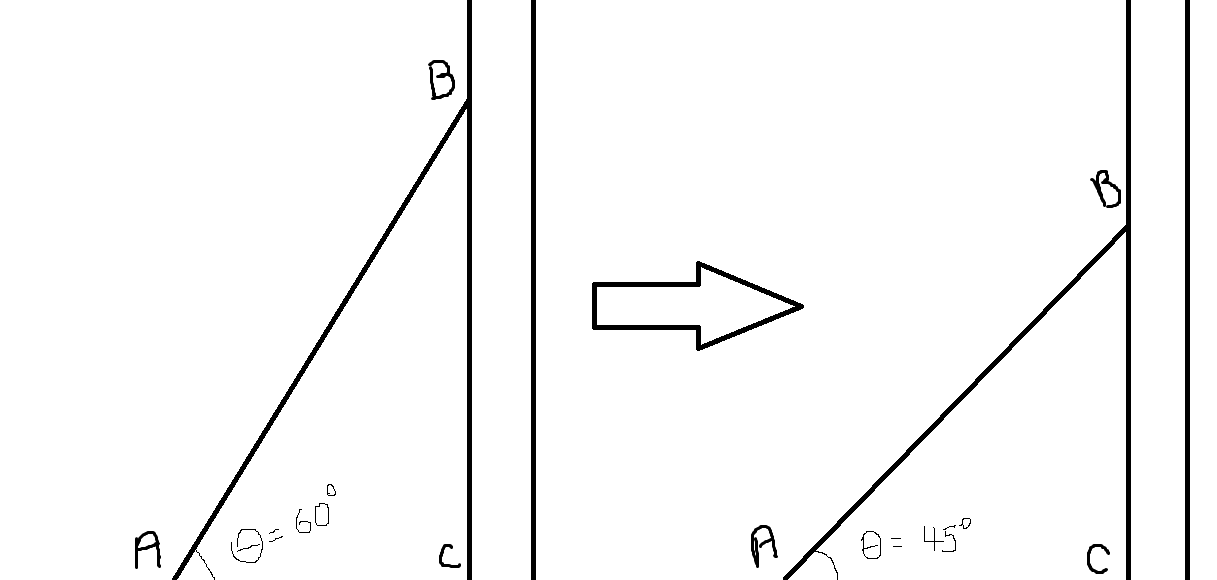
We know that in the first diagram, `theta=60^@` and `AB=8`.

We know that in the second diagram, `theta=45^@` and `AB=8` as the ladder's length does not change.

Using the trig ratio `sintheta=("opposite")/("hypotenuse")`

I can say in both diagrams, `sintheta=(BC)/(AB)`

In the first diagram, `sin60=(BC)/8`
`BC=8sin60`

In the second diagram, `sin45=(BC)/8`
`BC=8sin45`

Now, we can see that the change in height is just:
`8sin60-8sin45=8*sqrt(3)/2-8*1/sqrt(2)`
`=4sqrt(3)-4sqrt(2)`
`=4(sqrt(3)-sqrt(2))`

`DeltaBC=4(sqrt(3)-sqrt(2))`

As to where the values came from, there's a table for it.
If you are up to a challenge, you can try deriving where the exact values of the trig functions came from.

Answer 2

`sin60 = h/8`.....where h is the height of the top of the ladder from ground.

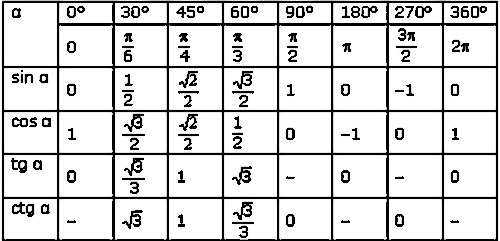
so, `h = 8sin60`

=> `h = 8(sqrt3)/2 => 4sqrt3`

When the ladder makes an angle of 45 degrees we have:

`sin45 = h/8`

i.e. `h = 8sin45`

=> `h = 8(sqrt2)/2 => 4sqrt2`

Hence, distance of slip is:

`4sqrt3 - 4sqrt2`

i.e. `4(sqrt3 - sqrt2)`

:)>