Question

Question #0bc37

Answer 1

I would say opposite.

Explanation:

Vectorially the velocity will be, say, towards the positive `x` while the acceleration will be in the opposite direction (negative `x`). This "opposition" will be "representative" of the reduction in modulus of the velocity. As long as it is operating, velocity will decrease and eventually go to to zero. If this deceleration contiues further the object will start to reverse its motion and now its velocity will start to increase in moduus but in the negative `x` direction!

The situation, when acceleration and velocity have the same direction, will result in an increas of the modulus of the velocity.

As an example consider a car that has a velocity of, say, `20m/s` and you apply the brakes.
Logically (and hopefully!) the velocity shoud decrease...so that the final velocity `v_f` (after a time `t` during which you apply the brakes) should depend upon the ACTION of the brakes...i.e. an acceleration `a`; so you have:
`v_f=v_i+at`
as we logically imagine the acceleration must have a "quality" that makes the velocity to decrease! Vectorially we say that the acceleration will oppose the velocity direction and in the formula it will appear a negative sign. If the initial velocity direction was positive the acceleration sign must be negative to ensure a reduction of velocity!
Say, that the acceleration is `-3m/s^2` operating for `2s` you'llget:
`v_f=20-3*2=20-6=14m/s` that it is indeed less!
Now, the brakes alone are not very good for the next example but imagine that you have a kind of "engine operated brake system" so that it keeps on accelerating (negatively) for how long as you like. Imagine that our system works now for `10s`; we will get:
`v_f=20-3*10=20-30=-10m/s`
the minus tells us that the final velocity is now in the opposite direction compared to the initial one (and the same as the acceleration) so that our car is now moving in the opposite direction!

Hope it helps!

Answer 2

See answer below.

Explanation:

Acceleration is the rate of change of velocity with respect to time:

`veca=(dv)/(dt)`

In other words, the acceleration is the gradient of the velocity vs time graph. Because it is a vector, direction is important. This means that a positive gradient is a positive acceleration, and a negative gradient is a negative acceleration.

Take the following three graphs. 1) Position vs time. 2) Velocity vs time. 3) Acceleration vs time.

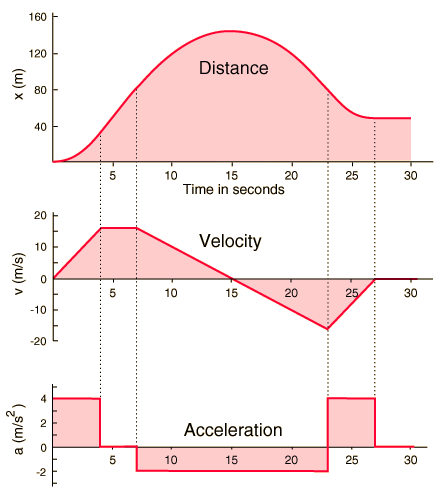
The acceleration's magnitude and direction is always given by the gradient of the velocity vs time graph.

If you increase velocity in the positive direction, acceleration is positive and in the same direction. This is seen from 0-4 s.
From 4-7 s, the velocity is constant and the gradient/acceleration is zero.

From 7-15 s, the velocity is still positive but decreasing. This means that the object is slowing down. The acceleration is in the opposite direction to the velocity, so it is negative and called deceleration.

At 15 s, the object stops and turns around. It is speeding up in the negative direction/going back to where it started, which means the velocity is negative. It is accelerating too, but in the negative direction, so has a negative acceleration.

At 23 s, the object starts braking again but because the gradient is positive, acceleration is positive. So, from 23-27 s, the velocity is negative, but getting less negative until the object stops at 27 s. The acceleration is slowing the object down, so it must be acting in the opposite/positive direction.

Answer 3

Though the two answers have explained the concept quite clearly, I will put my answer as asked.

Explanation:

Newton's First Law of Motion states that an object in the state of rest or of uniform motion continues to stay in the same state unless acted upon by an unbalanced force.

We understand from this Law that a Force is necessary to change the state of rest or of uniform motion of an object.

Newton's Second Law of Motion quantifies how much change a force will produce.

Secondly it must be borne in mind that all quantities like Force, Acceleration, Velocity and Displacement are vector quantities. As such each has associated magnitude and direction. Many a times in equations we do omit placement of arrow at the top `vecF`, for sake of convenience, and write

`F=ma`.

Actually it must always be taken as

`vecF=mveca`

Lastly, you are free to select your own coordinate system to define origin and directions in a particular problem. A word of caution, we select a specific coordinate system to make problem and its solution look simpler and easy. Hence, it is always a good idea to select the coordinate system as many others do and is as shown below.

Armed with basics we return to your question.

1. The vehicle is moving with velocity `vecv`. Suppose it is in `+x` direction.
2. Q.: Direction of acceleration with respect to velocity when velocity decreases?
   We note that velocity is decreasing on the application of brakes. This can happen only if the force acts in a direction opposite to the direction of motion. We therefore, come to conclusion that acceleration produced by the applied force (brakes in this question) must be in a direction opposite to the direction of motion. Which gives us direction of acceleration `veca` as `-x`

You also want to know the direction of acceleration with respect to velocity when velocity is increasing.

Consider an object initially at rest and acted upon by a force. The object will start moving in the direction of applied force and its velocity will increase in the same direction.

For an object already moving with velocity `vecv`, we see that velocity can increase only if applied force (or acceleration `veca`) is in the direction of motion.

Hope this helps.