Question

Physics Question: A 2.0 kg block is launched up a frictionless ramp?

A 2.0 kg block is launched up a frictionless ramp that is inclined at 35 degrees. The block's initial speed is 10 m/s.
What vertical height does the block reach above its starting point?
What speed does it have when it slides back down to its starting point?

Answer 1

`d_"vertical"=5.1" "m`

`"speed"=10" "m/s`

Explanation:

The easiest way to find the answer for the speed is to know that under constant acceleration for a projectile (the acceleration is the same going up as it is coming down), the speed when the object gets back to its starting position will be the same as its initial speed. So the answer is `10" "m/s`.

The equations of motion will show you that the time taken to go up is equal to the time taken to come down, the distance travelled is the same both ways and the final speed will be the same as the initial speed. To use the equations, break the motion into two sections, upwards and downwards, and solve separately.

But we need the acceleration, which is the net force parallel to the slope. There are two forces acting on the block, the normal force from the surface, `F_N` and the weight force, `F_g`. As the block is accelerating, these forces are unbalanced and there is a net force down the slope, `F_"net"`.

If we break the weight force into its two components (see pic), it has a parallel and a perpendicular component, relative to the surface. As there is no motion perpendicular to the slope, the normal force is equal to the perpendicular component of the weight force, but the parallel component will give the net force down the slope.

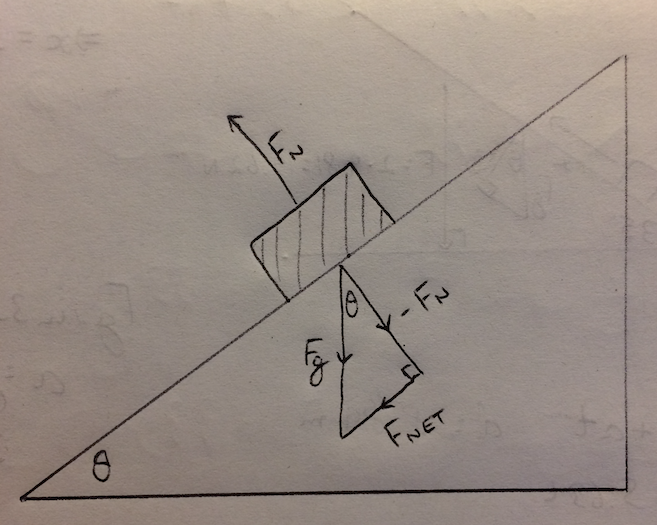
Using trigonometry, `F_"net"` is given by:

`F_"net"=F_gsin(theta)`

Now we can find the acceleration:

`F_"net"=F_gsin(theta)`

`rArrma=mgsin(theta)`

`rArra=gsin(theta)=9.8sin(35)=5.6" "m/s^2`

Now use an equation of motion to solve for the distance travelled up the slope:

`v^2=u^2+2ad`

`v=0" "m/s`
`u=10" "m/s`
`a=-5.6" "m/s^2`

`d=(v^2-u^2)/(2a)`

`d=(0^2-10^2)/(2*-5.6)=8.9" "m`

If we look at the downward motion separately and make this the positive direction for simplicity, we know that the distance travelled back down the slope is the same and that the acceleration is the same. So the final speed will be:

`v_2^2=u_2^2+2ad`

`u_2=0" "m/s`

`a=5.6" "m/s^2`

`d=8.9" "m`

`v_2^2=0^2+2*5.6*8.9=99.7`

`rArrv_2=sqrt(99.7)~~10" "m/s`

It's 10 m/s, just like we predicted, but with rounding errors.

For the vertical height, set up a right-angled triangle and substitute in the value for `d` as the hypotenuse and 35˚ for theta:

`d_"vertical"=dsin(35)=8.9sin(35)=5.1" "m`