How do you solve the #triangle ABC# given #A=50^circ, a=2.5, C=67^circ#?

Question

2061 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-08-05T19:55:56

See below.

Since all the angles of a triangle sum to #180^@#, we can say

#m /_ A + m /_ B + m /_ C = 180^@#

Substitute in the given information and solve for #m/_B#:

#50^@ + m /_ B + 67^@ = 180^@#

#m /_ B + 117^@ = 180^@#

#m /_ B = 180^@ - 117^@#

#m /_ B = 63^@#

We now have the following triangle:

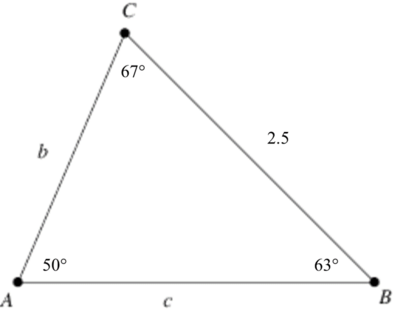
The Law of Sines states that #sin A/a = sin B / b = sin C / c#.

Thus, we can say

#sin 50^@/2.5 = sin 63^@/b#

Cross-multiply and solve for #b#:

#b * sin 50^@ = 2.5 * sin 63^@#

#b = (2.5 * sin 63^@)/sin 50^@#

#b=2.9#

Do the same to find #c#:

#sin 50^@/2.5 = sin 67^@/c#

#c * sin 50^@ = 2.5 * sin 67^@#

#c = (2.5 * sin 67^@)/sin 50^@#

#c=3.0#

#m/_A=50^@#
#m/_B=63^@#
#m/_C=67^@#

#a=2.5#
#b=2.9#
#c=3.0#