What is the equation in standard form of the parabola with a focus at (-2,3) and a directrix of y= -9?
1 Answer
Explanation:
Sketch the directrix and focus (point
Choose a general point on the parabola (called
Join
A horizontal line from
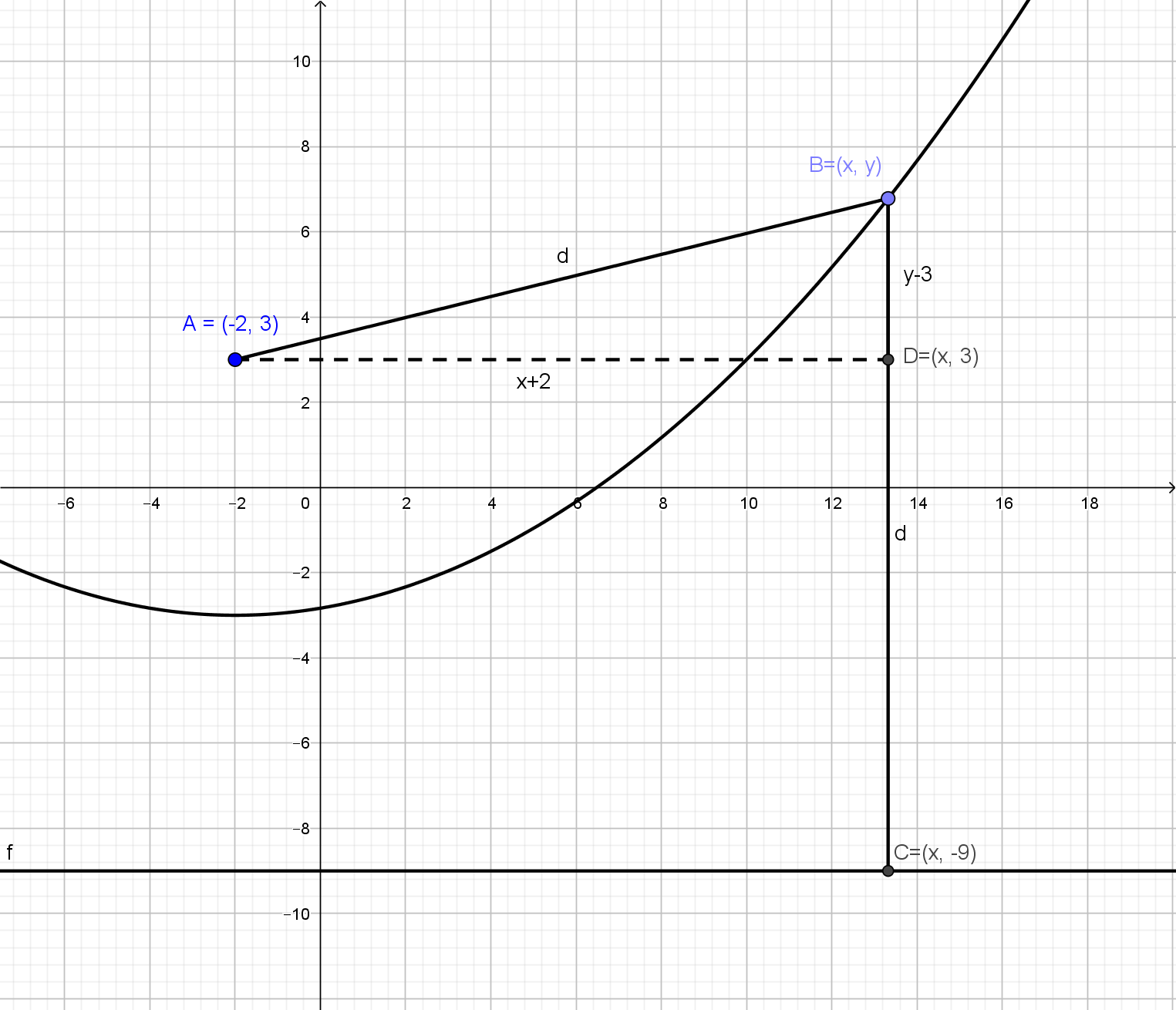
By the parabola definition, point
Find expressions for the distances
Then use Pythagoras to find AB:
and since
This is your parabola equation.
If you want it in explicit

