How is the answer -2/3 and -4/3? I do not understand how this can be.
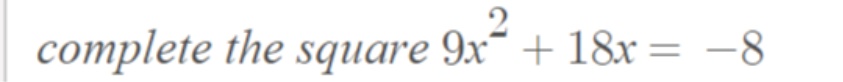
2 Answers
Read the full explanation below :)
Explanation:
First, set your equation equal to zero.
Now, we simply use the quadratic formula.
First, solve for your discriminant, the portion marked in blue. Recall that a quadratic is written as
Now, plug that into the quadratic formula.
Plug in the rest of your numbers.
Simplify.
Solve.
Explanation:
#"using the method of "color(blue)"completing the square"#
#y=9x^2+18x+8=0#
#• " the coefficient of the "x^2" term must be 1"#
#rArr9(x^2+2x+8/9)=0#
#• "add/subtract "(1/2"coefficient of x-term")^2" to "x^2+2x#
#rArr9(x^2+2(1)xcolor(red)(+1)color(red)(-1)+8/9)=0#
#rArr9(x+1)^2+9(-1+8/9)=0#
#rArr9(x+1)^2-1=0#
#rArr(x+1)^2=1/9#
#color(blue)"take the square root of both sides"#
#rArrx+1=+-sqrt(1/9)larrcolor(blue)"note plus or minus"#
#rArrx=-1+-1/3#
#rArrx=-1-1/3=-4/3" or "x=-1+1/3=-2/3#

