Question

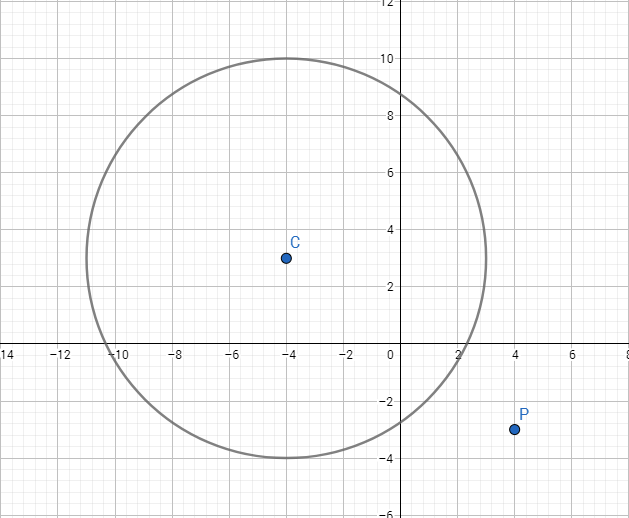
Does the point `(4,-3)` lie on the circle `(x+4)^2+(y-3)^2=49`?

Answer 1

No, it does't lie on the circle.

Explanation:

The equation of a circle is given by:
`(x-a)^2+(y-b)^2=r^2`, where:

• `a` is the `y` co-ordinate of the center of the circle
• `b` is the `y` co-ordinate of the center of the circle
• `r` is the radius of the circle

Using the given information we can get:
`(x+4)^2+(y-3)^2=7^2=49`

Using the the point `(4,-3)=>(x,y)` we get:
`(4+4)^2+(-3-3)^2=8^2+(-6)^2=64+36=100`

`sqrt(100)=10!=7`

Answer 2

No, it does not.

Explanation:

For the point `(4,-3)` to lie on the circle, its distance from the centre must be exactly `7` units.

To find the distance between the point and the centre, use Pythagoras Theorem,

Distance`=sqrt((4-(-4))^2+(-3-3)^2)`
`color(white)(xxxx/x.)=sqrt100`
`color(white)(xxxx/x.)=10`

Since the distance from of the point from the centre is more than `7`, the point lies outside of the circle and does not lie on the circle.

Check: