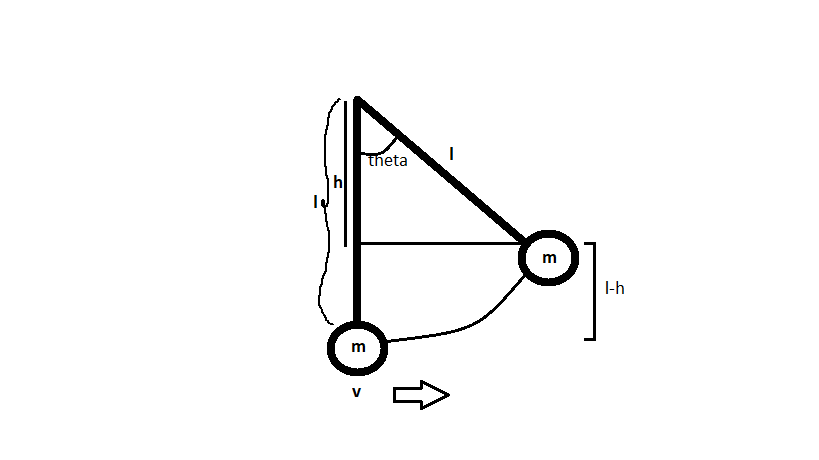
See the diagram made,where a pendulum of string length #l# and a bob of mass #m# reaches up to the shown position from its mean point,and at the mean position, it had a velocity of #v#
So,vertically it has shifted a distance of #(l-h)# (from the diagram)
Now, #h/l = cos theta# or, #h = l cos theta# ,so #(l-h) = l(1-cos theta)#
Now, in its pathway,energy will be conserved.
So,at its mean position,total energy is kinetic energy i.e #1/2mv^2#
And, at the highest point,its total energy is purely potential energy i.e #mg(l-h)# i.e #mgl(1-cos theta)#
So,equating both we get,
#v=sqrt(2gl(1-cos theta)#
ALTERNATIVELY,
Suppose, a particle in S.H.M follows the equation,
#x= a sin omegat# ......1 (here,#a# is the amplitude of its motion)
For,a simple pendulum, #omega = sqrt(g/l)#,where #g# is acceleration due to gravity and #l# is the length of the pendulum)
So, its velocity equation will be, #v=aomega cos omegat# (by differentiating 1, as #v=(dx)/dt#
From 1 we can say #cos omegat = sqrt(1-(x/a)^2)# (as #sin^2omegat+cos^2omegat=1#)
So,putting the value of #cosomegat# in the velocity equation,we get,
#v=omegasqrt(a^2-x^2)# ....2
Now, for the given equation, #x=asinomegat#, mean position is at #x=0#
So,putting #x=0# in equation 2 we get, #v=omegaa#
This the equation of the velocity of a particle under SHM at its mean position.
Now, see maximum value of #v# in the equation 2 will come,when #x# will be zero,so maximum value of #v# becomes #omegaa#
That means,velocity is the maximum in the mean position as well.


