Why is the function not differentiable?
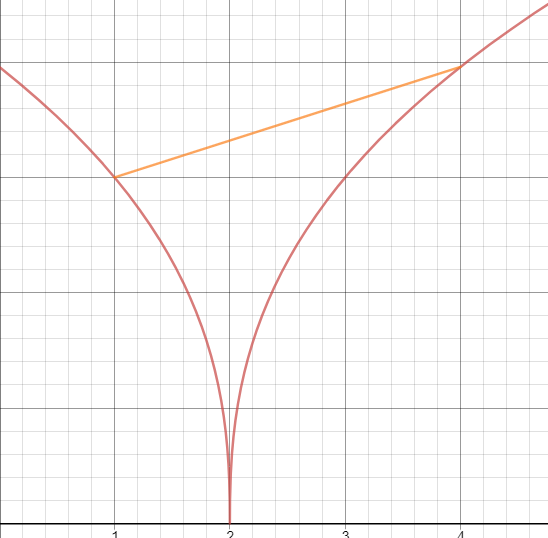
Consider the function f(x) = 3(x - 2)^2/5
A)The function is not differentiable at x = 2. Why not?
B)Will the Mean Value Theorem apply in the interval [2, 5] even though the function is not differentiable at x = 2? Explain.
C) Will the Mean Value Theorem apply to the interval [1, 4]? Explain.
Consider the function f(x) = 3(x - 2)^2/5
A)The function is not differentiable at x = 2. Why not?
B)Will the Mean Value Theorem apply in the interval [2, 5] even though the function is not differentiable at x = 2? Explain.
C) Will the Mean Value Theorem apply to the interval [1, 4]? Explain.
1 Answer
Explanation:
Question A
You can see this multiple different ways. Either we can differentiate the function to find:
which is undefined at
Or, we can look at the limit:
This limit limit doesn't exist, which means that the derivative doesn't exist in that point.
Question B
Yes, the Mean Value Theorem does apply. The differentiability condition in the Mean Value Theorem only requires the function to be differentiable on the open interval
We can also see that there is indeed a point with the average slope in that interval:

Question C
No. As previously mentioned, the Mean Value Theorem requires the function to be entirely differentiable on the open interval
We can also see that there is no point in the interval which contains the average slope on this function, because of the "sharp bend" in the curve.