Question

How do you solve `10= 2e^ { 5x }`?

Answer 1

`x~~0.322" to 3 dec. places"`

Explanation:

`"using the "color(blue)"law of logarithms"`

`•color(white)(x)logx^nhArrnlogx`

`"divide both sides by 2"`

`rArre^(5x)=10/2=5`

`"take the ln (natural log) of both sides"`

`rArrlne^(5x)=ln5`

`rArr5xcancel(lne)^1=ln5`

`rArrx=ln5/5~~0.322" to 3 dec. places"`

Answer 2

x=ln(5)/5

Explanation:

`10=2e^(5x)`
or `5=e^(5x)`
Take natural log on both sides
`ln(5)=ln(e^(5x))`

Using property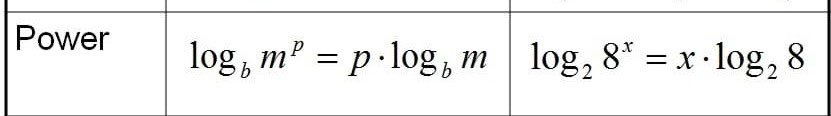

`ln(5)=5x*ln(e)`
0r
ln(5)=5x [since ln(e)=1 ]

x=ln(5)/5