Question

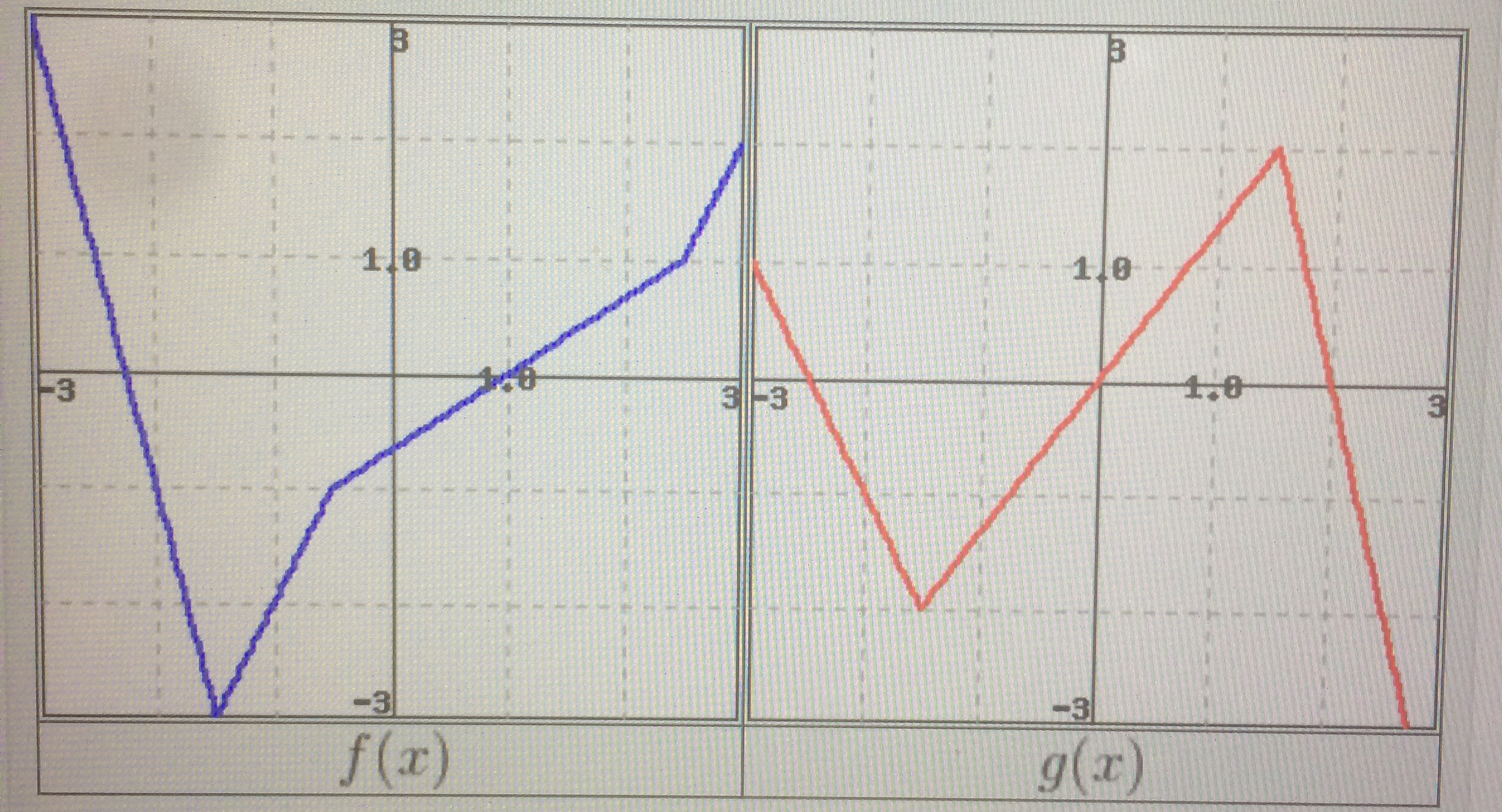
Let h(x)=f(x)*g(x), and k(x)=f(x)/g(x). Use the figures below to find the exact values of the indicated derivatives? h'(-1) and k'(1)?

Answer 1

`h'(-1)=-16/3`

Explanation:

The product rule states, if `h(x)=f(x)g(x)`, then

`h'(x)=f'(x)g(x)+f(x)g'(x)`

We are ask to find `h'(-1)`, or by the product rule

`h'(-1)=f'(-1)g(-1)+f(-1)g'(-1)`

The values of the functions must be

`f(-1)=-2` and `g(-1)=-4/3`

Remember the derivative gives the slope of any given point,
but as we can see in the figures these must correspond,
to the slope of the line, which goes through the point, thus

`f'(-1)=(Deltay)/(Deltax)=2/1=2`

And

`g'(-1)=(Deltay)/(Deltax)=4/3`

We can conclude

`h'(-1)=2*(-4/3)+4/3(-2)=-16/3`

The other exercise, to find `k'(-1)`, follows a very similar approach,
i will leave it for you, feel free to ask if anything is unclear